Improved subsample-and-aggregate via the private modified winsorized mean

We develop a univariate, differentially private mean estimator, called the private modified winsorized mean designed to be used as the aggregator in subsample-and-aggregate. We demonstrate, via real data analysis, that common differentially private multivariate mean estimators may not perform well as the aggregator, even with a dataset with 8000 observations, motivating our developments. We show that the modified winsorized mean is minimax optimal for several, large classes of distributions, even under adversarial contamination. We also demonstrate that, empirically, the modified winsorized mean performs well compared to other private mean estimates. We consider the modified winsorized mean as the aggregator in subsample-and-aggregate, deriving a finite sample deviations bound for a subsample-and-aggregate estimate generated with the new aggregator. This result yields two important insights: (i) the optimal choice of subsamples depends on the bias of the estimator computed on the subsamples, and (ii) the rate of convergence of the subsample-and-aggregate estimator depends on the robustness of the estimator computed on the subsamples.

Differentially private projection-depth-based medians

We develop $(\epsilon,\delta)$-differentially private projection-depth-based medians using the propose-test-release (PTR) and exponential mechanisms. Under general conditions on the input parameters and the population measure, (e.g. we do not assume any moment bounds), we quantify the probability the test in PTR fails, as well as the cost of privacy via finite sample deviation bounds. We demonstrate our main result on the canonical projection-depth-based median. In the Gaussian setting, we show that the resulting deviation bound matches the known lower bound for private Gaussian mean estimation, up to a polynomial function of the condition number of the covariance matrix. In the Cauchy setting, we show that the ``outlier error amplification'' effect resulting from the heavy tails outweighs the cost of privacy. This result is then verified via numerical simulations. Additionally, we present results on general PTR mechanisms and a uniform concentration result on the projected spacings of order statistics.

Concentration of the exponential mechanism and differentially private multivariate medians

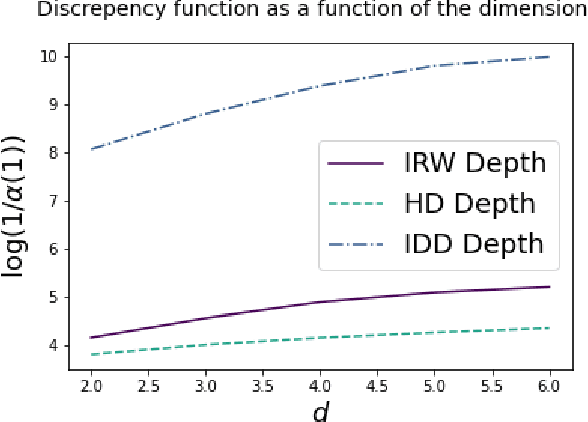
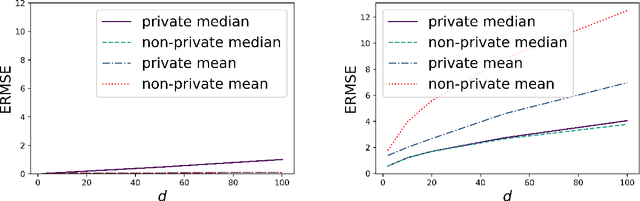
We prove concentration inequalities for the output of the exponential mechanism about the maximizer of the population objective function. This bound applies to objective functions that satisfy a mild regularity condition. To illustrate our result, we study the problem of differentially private multivariate median estimation. We present novel finite-sample performance guarantees for differentially private multivariate depth-based medians which are essentially sharp. Our results cover commonly used depth functions, such as the halfspace (or Tukey) depth, spatial depth, and the integrated dual depth. We show that under Cauchy marginals, the cost of heavy-tailed location estimation outweighs the cost of privacy. We demonstrate our results numerically using a Gaussian contamination model in dimensions up to $d = 100$, and compare them to a state-of-the-art private mean estimation algorithm.