Jonas Skackauskas
Herder Ants: Ant Colony Optimization with Aphids for Discrete Event-Triggered Dynamic Optimization Problems
Apr 15, 2023Abstract:Currently available dynamic optimization strategies for Ant Colony Optimization (ACO) algorithm offer a trade-off of slower algorithm convergence or significant penalty to solution quality after each dynamic change occurs. This paper proposes a discrete dynamic optimization strategy called Ant Colony Optimization (ACO) with Aphids, modelled after a real-world symbiotic relationship between ants and aphids. ACO with Aphids strategy is designed to improve solution quality of discrete domain Dynamic Optimization Problems (DOPs) with event-triggered discrete dynamism. The proposed strategy aims to improve the inter-state convergence rate throughout the entire dynamic optimization. It does so by minimizing the fitness penalty and maximizing the convergence speed that occurs after the dynamic change. This strategy is tested against Full-Restart and Pheromone-Sharing strategies implemented on the same ACO core algorithm solving Dynamic Multidimensional Knapsack Problem (DMKP) benchmarks. ACO with Aphids has demonstrated superior performance over the Pheromone-Sharing strategy in every test on average gap reduced by 29.2%. Also, ACO with Aphids has outperformed the Full-Restart strategy for large datasets groups, and the overall average gap is reduced by 52.5%.
Dynamic Impact for Ant Colony Optimization algorithm
Feb 10, 2020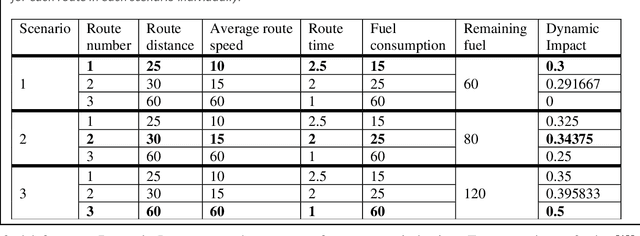
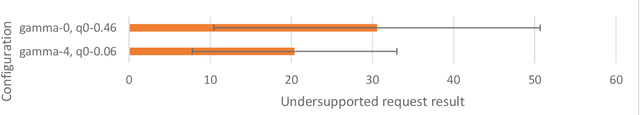
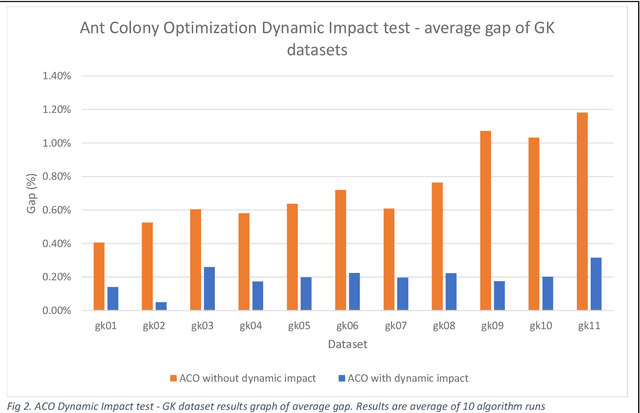
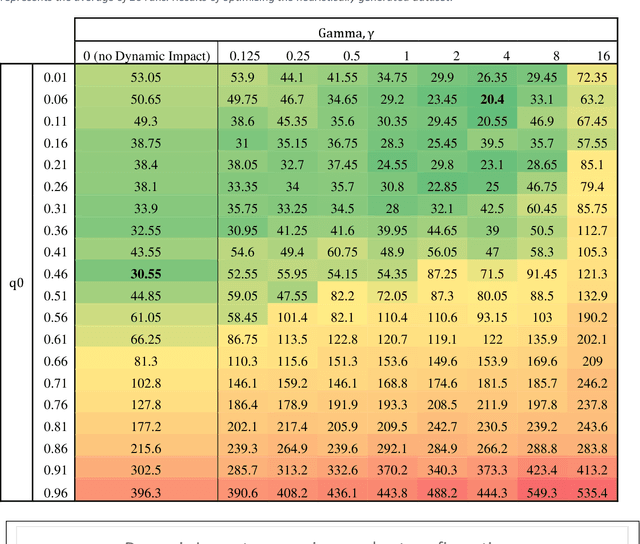
Abstract:This paper proposes an extension method for Ant Colony Optimization (ACO) algorithm called Dynamic Impact. Dynamic Impact is designed to solve challenging optimization problems that has nonlinear relationship between resource consumption and fitness in relation to other part of the optimized solution. This proposed method is tested against complex real-world Microchip Manufacturing Plant Production Floor Optimization (MMPPFO) problem, as well as theoretical benchmark Multi-Dimensional Knapsack problem (MKP). MMPPFO is a non-trivial optimization problem, due the nature of solution fitness value dependence on collection of wafer-lots without prioritization of any individual wafer-lot. Using Dynamic Impact on single objective optimization fitness value is improved by 33.2%. Furthermore, MKP benchmark instances of small complexity have been solved to 100% success rate where high degree of solution sparseness is observed, and large instances have showed average gap improved by 4.26 times. Algorithm implementation demonstrated superior performance across small and large datasets and sparse optimization problems.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge