Model Transformations for Ranking Functions and Total Preorders

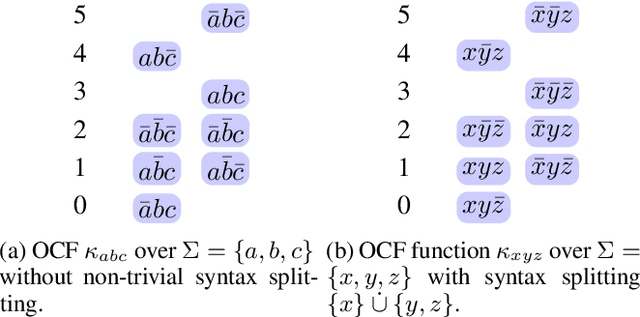
In the field of knowledge representation, the considered epistemic states are often based on propositional interpretations, also called worlds. E.g., epistemic states of agents can be modelled by ranking functions or total preorders on worlds. However, there are usually different ways of how to describe a real world situation in a propositional language; this can be seen as different points of view on the same situation. In this paper we introduce the concept of model transformations to convert an epistemic state from one point of view to another point of view, yielding a novel notion of equivalence of epistemic states. We show how the well-known advantages of syntax splitting, originally developed for belief sets and later extended to representation of epistemic states and to nonmonotonic reasoning, can be exploited for belief revision via model transformation by uncovering splittings not being present before. Furthermore, we characterize situations where belief change operators commute with model transformations.

Inference with System W Satisfies Syntax Splitting

In this paper, we investigate inductive inference with system W from conditional belief bases with respect to syntax splitting. The concept of syntax splitting for inductive inference states that inferences about independent parts of the signature should not affect each other. This was captured in work by Kern-Isberner, Beierle, and Brewka in the form of postulates for inductive inference operators expressing syntax splitting as a combination of relevance and independence; it was also shown that c-inference fulfils syntax splitting, while system P inference and system Z both fail to satisfy it. System W is a recently introduced inference system for nonmonotonic reasoning that captures and properly extends system Z as well as c-inference. We show that system W fulfils the syntax splitting postulates for inductive inference operators by showing that it satisfies the required properties of relevance and independence. This makes system W another inference operator besides c-inference that fully complies with syntax splitting, while in contrast to c-inference, also extending rational closure.

Descriptor Revision for Conditionals: Literal Descriptors and Conditional Preservation

Descriptor revision by Hansson is a framework for addressing the problem of belief change. In descriptor revision, different kinds of change processes are dealt with in a joint framework. Individual change requirements are qualified by specific success conditions expressed by a belief descriptor, and belief descriptors can be combined by logical connectives. This is in contrast to the currently dominating AGM paradigm shaped by Alchourr\'on, G\"ardenfors, and Makinson, where different kinds of changes, like a revision or a contraction, are dealt with separately. In this article, we investigate the realisation of descriptor revision for a conditional logic while restricting descriptors to the conjunction of literal descriptors. We apply the principle of conditional preservation developed by Kern-Isberner to descriptor revision for conditionals, show how descriptor revision for conditionals under these restrictions can be characterised by a constraint satisfaction problem, and implement it using constraint logic programming. Since our conditional logic subsumes propositional logic, our approach also realises descriptor revision for propositional logic.