Spatial-Frequency Domain Nonlocal Total Variation for Image Denoising

Following the pioneering works of Rudin, Osher and Fatemi on total variation (TV) and of Buades, Coll and Morel on non-local means (NL-means), the last decade has seen a large number of denoising methods mixing these two approaches, starting with the nonlocal total variation (NLTV) model. The present article proposes an analysis of the NLTV model for image denoising as well as a number of improvements, the most important of which being to apply the denoising both in the space domain and in the Fourier domain, in order to exploit the complementarity of the representation of image data in both domains. A local version obtained by a regionwise implementation followed by an aggregation process, called Local Spatial-Frequency NLTV (L- SFNLTV) model, is finally proposed as a new reference algorithm for image denoising among the family of approaches mixing TV and NL operators. The experiments show the great performance of L-SFNLTV, both in terms of image quality and of computational speed, comparing with other recently proposed NLTV-related methods.

A note on patch-based low-rank minimization for fast image denoising

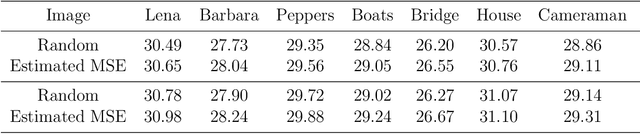
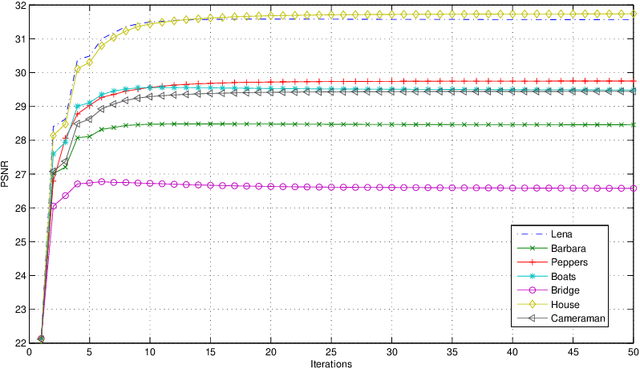
Patch-based low-rank minimization for image processing attracts much attention in recent years. The minimization of the matrix rank coupled with the Frobenius norm data fidelity can be solved by the hard thresholding filter with principle component analysis (PCA) or singular value decomposition (SVD). Based on this idea, we propose a patch-based low-rank minimization method for image denoising. The main denoising process is stated in three equivalent way: PCA, SVD and low-rank minimization. Compared to recent patch-based sparse representation methods, experiments demonstrate that the proposed method is rather rapid, and it is effective for a variety of natural grayscale images and color images, especially for texture parts in images. Further improvements of this method are also given. In addition, due to the simplicity of this method, we could provide an explanation of the choice of the threshold parameter, estimation of PSNR values, and give other insights into this method.