Investigating and Mitigating Barren Plateaus in Variational Quantum Circuits: A Survey

In recent years, variational quantum circuits (VQCs) have been widely explored to advance quantum circuits against classic models on various domains, such as quantum chemistry and quantum machine learning. Similar to classic machine-learning models, VQCs can be optimized through gradient-based approaches. However, the gradient variance of VQCs may dramatically vanish as the number of qubits or layers increases. This issue, a.k.a. Barren Plateaus (BPs), seriously hinders the scaling of VQCs on large datasets. To mitigate the exponential gradient vanishing, extensive efforts have been devoted to tackling this issue through diverse strategies. In this survey, we conduct a systematic literature review of recent works from both investigation and mitigation perspectives. Besides, we propose a new taxonomy to categorize most existing mitigation strategies. At last, we provide insightful discussion for future directions of BPs.

Improving Trainability of Variational Quantum Circuits via Regularization Strategies

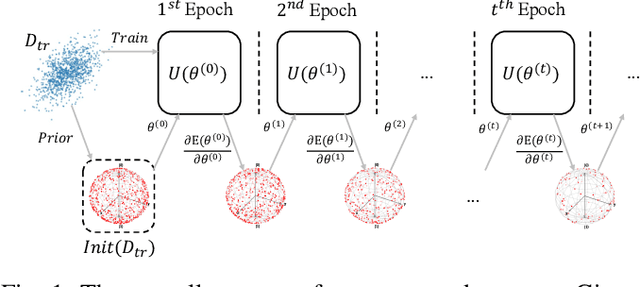
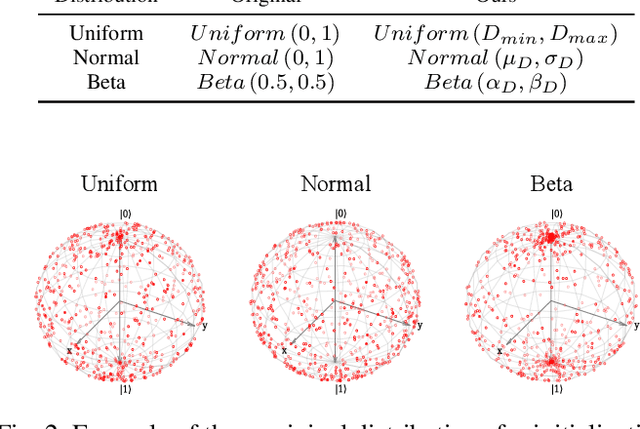
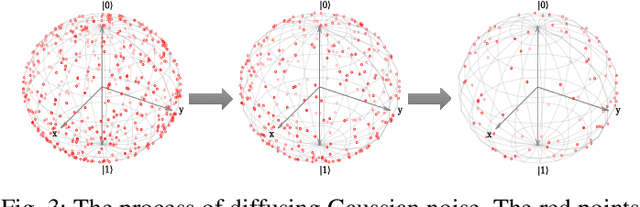
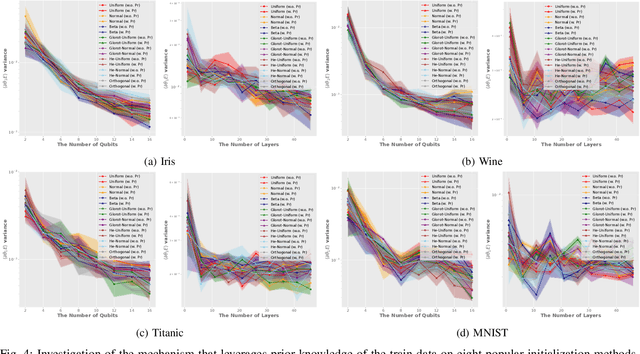
In the era of noisy intermediate-scale quantum (NISQ), variational quantum circuits (VQCs) have been widely applied in various domains, advancing the superiority of quantum circuits against classic models. Similar to classic models, regular VQCs can be optimized by various gradient-based methods. However, the optimization may be initially trapped in barren plateaus or eventually entangled in saddle points during training. These gradient issues can significantly undermine the trainability of VQC. In this work, we propose a strategy that regularizes model parameters with prior knowledge of the train data and Gaussian noise diffusion. We conduct ablation studies to verify the effectiveness of our strategy across four public datasets and demonstrate that our method can improve the trainability of VQCs against the above-mentioned gradient issues.