Group-Control Motion Planning Framework for Microrobot Swarms in a Global Field

This paper investigates how group-control can be effectively used for motion planning for microrobot swarms in a global field. We prove that Small-Time Local Controllability (STLC) in robot positions is achievable through group-control, with the minimum number of groups required for STLC being $\log_2(n + 2) + 1$ for $n$ robots. We then discuss the complexity trade-offs between control and motion planning. We show how motion planning can be simplified if appropriate primitives can be achieved through more complex control actions. We identify motion planning problems that balance the number of robot groups and motion primitives with planning complexity. Various instantiations of these motion planning problems are explored, with simulations to demonstrate the effectiveness of group-control.

Group-based control of large-scale micro-robot swarms with on-board Physical Finite-State Machines

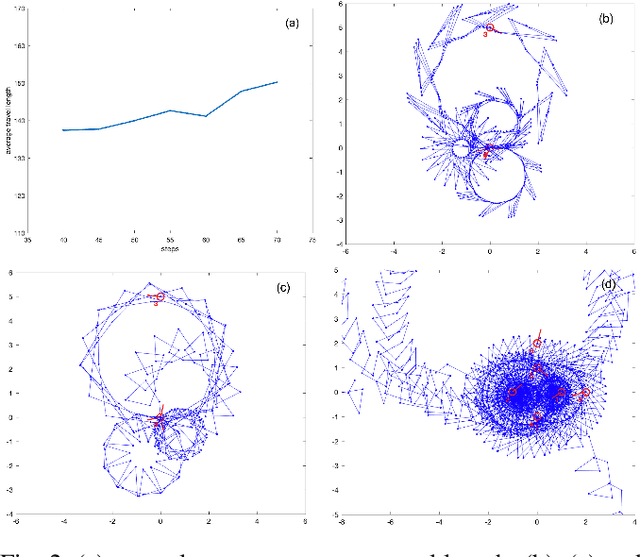
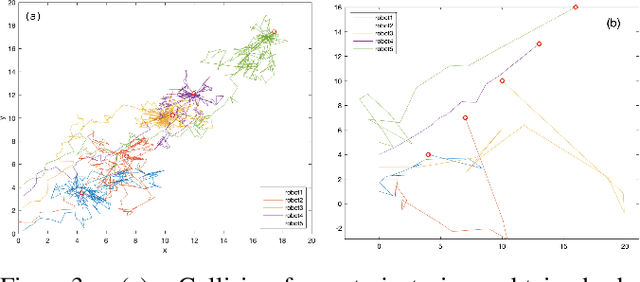
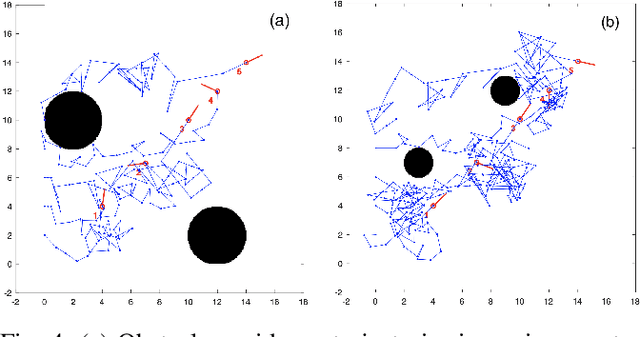
An important problem in microrobotics is how to control a large group of microrobots with a global control signal. This paper focuses on controlling a large-scale swarm of MicroStressBots with on-board physical finite-state machines. We introduce the concept of group-based control, which makes it possible to scale up the swarm size while reducing the complexity both of robot fabrication as well as swarm control. We prove that the group-based control system is locally accessible in terms of the robot positions. We further hypothesize based on extensive simulations that the system is globally controllable. A nonlinear optimization strategy is proposed to control the swarm by minimizing control effort. We also propose a probabilistically complete collision avoidance method that is suitable for online use. The paper concludes with an evaluation of the proposed methods in simulations.