Computing the Distance between unbalanced Distributions -- The flat Metric

We provide an implementation to compute the flat metric in any dimension. The flat metric, also called dual bounded Lipschitz distance, generalizes the well-known Wasserstein distance W1 to the case that the distributions are of unequal total mass. This is of particular interest for unbalanced optimal transport tasks and for the analysis of data distributions where the sample size is important or normalization is not possible. The core of the method is based on a neural network to determine on optimal test function realizing the distance between two given measures. Special focus was put on achieving comparability of pairwise computed distances from independently trained networks. We tested the quality of the output in several experiments where ground truth was available as well as with simulated data.

Dynamics of Pedestrian Crossing Decisions Based on Vehicle Trajectories in Large-Scale Simulated and Real-World Data

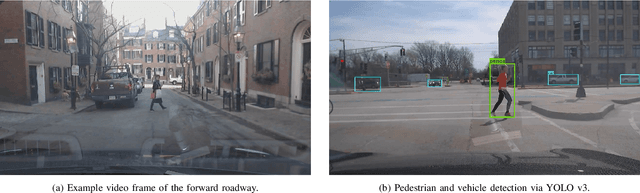
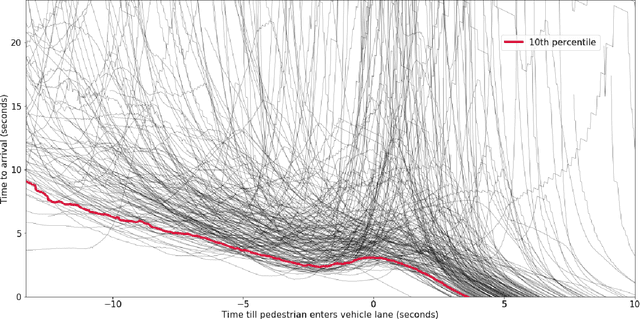
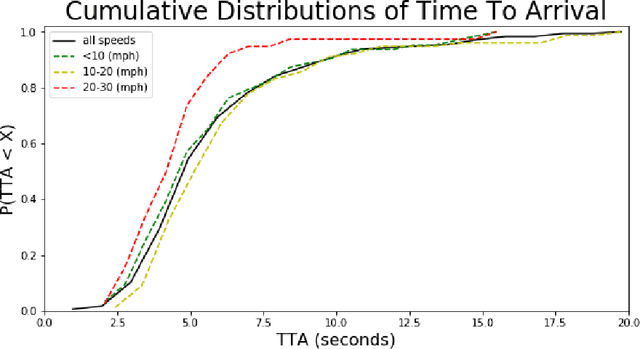
Humans, as both pedestrians and drivers, generally skillfully navigate traffic intersections. Despite the uncertainty, danger, and the non-verbal nature of communication commonly found in these interactions, there are surprisingly few collisions considering the total number of interactions. As the role of automation technology in vehicles grows, it becomes increasingly critical to understand the relationship between pedestrian and driver behavior: how pedestrians perceive the actions of a vehicle/driver and how pedestrians make crossing decisions. The relationship between time-to-arrival (TTA) and pedestrian gap acceptance (i.e., whether a pedestrian chooses to cross under a given window of time to cross) has been extensively investigated. However, the dynamic nature of vehicle trajectories in the context of non-verbal communication has not been systematically explored. Our work provides evidence that trajectory dynamics, such as changes in TTA, can be powerful signals in the non-verbal communication between drivers and pedestrians. Moreover, we investigate these effects in both simulated and real-world datasets, both larger than have previously been considered in literature to the best of our knowledge.