GraphTTA: Test Time Adaptation on Graph Neural Networks

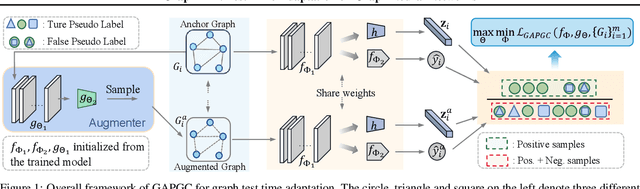
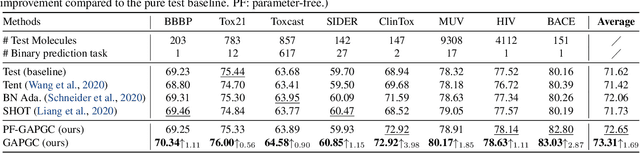
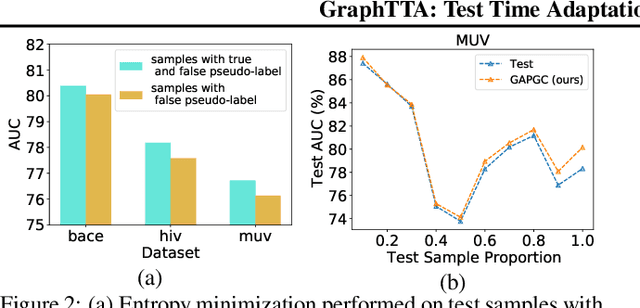
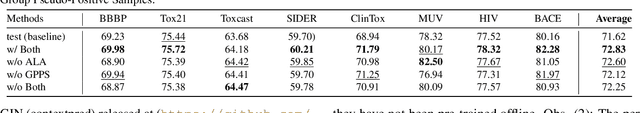
Recently, test time adaptation (TTA) has attracted increasing attention due to its power of handling the distribution shift issue in the real world. Unlike what has been developed for convolutional neural networks (CNNs) for image data, TTA is less explored for Graph Neural Networks (GNNs). There is still a lack of efficient algorithms tailored for graphs with irregular structures. In this paper, we present a novel test time adaptation strategy named Graph Adversarial Pseudo Group Contrast (GAPGC), for graph neural networks TTA, to better adapt to the Out Of Distribution (OOD) test data. Specifically, GAPGC employs a contrastive learning variant as a self-supervised task during TTA, equipped with Adversarial Learnable Augmenter and Group Pseudo-Positive Samples to enhance the relevance between the self-supervised task and the main task, boosting the performance of the main task. Furthermore, we provide theoretical evidence that GAPGC can extract minimal sufficient information for the main task from information theory perspective. Extensive experiments on molecular scaffold OOD dataset demonstrated that the proposed approach achieves state-of-the-art performance on GNNs.

Preventing Over-Smoothing for Hypergraph Neural Networks

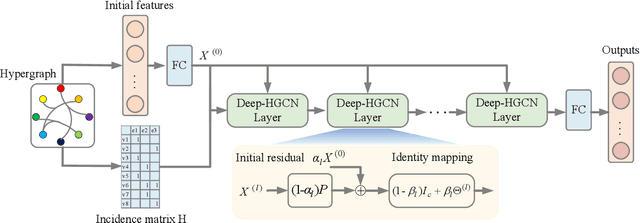
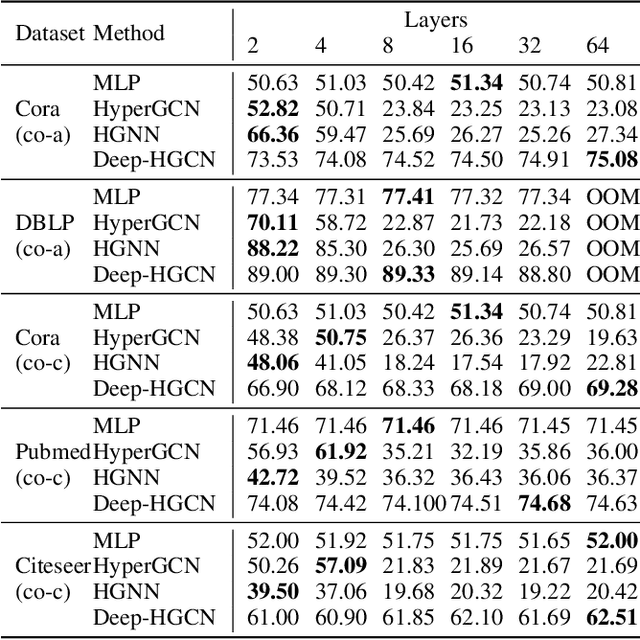
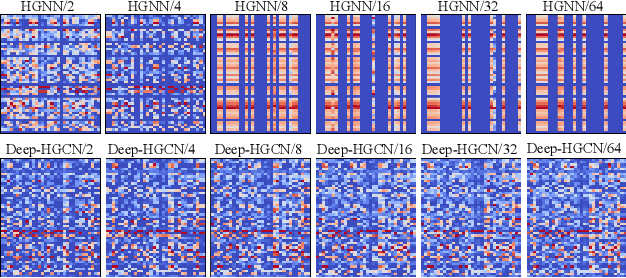
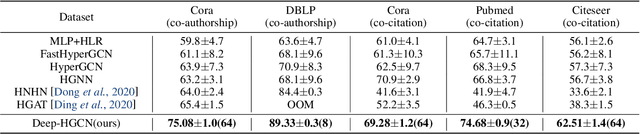
In recent years, hypergraph learning has attracted great attention due to its capacity in representing complex and high-order relationships. However, current neural network approaches designed for hypergraphs are mostly shallow, thus limiting their ability to extract information from high-order neighbors. In this paper, we show both theoretically and empirically, that the performance of hypergraph neural networks does not improve as the number of layers increases, which is known as the over-smoothing problem. To tackle this issue, we develop a new deep hypergraph convolutional network called Deep-HGCN, which can maintain the heterogeneity of node representation in deep layers. Specifically, we prove that a $k$-layer Deep-HGCN simulates a polynomial filter of order $k$ with arbitrary coefficients, which can relieve the problem of over-smoothing. Experimental results on various datasets demonstrate the superior performance of the proposed model comparing to the state-of-the-art hypergraph learning approaches.