Fluid-Antenna-aided AAV Secure Communications in Eavesdropper Uncertain Location

For autonomous aerial vehicle (AAV) secure communications, traditional designs based on fixed position antenna (FPA) lack sufficient spatial degrees of freedom (DoF), which leaves the line-of-sight-dominated AAV links vulnerable to eavesdropping. To overcome this problem, this paper proposes a framework that effectively incorporates the fluid antenna (FA) and the artificial noise (AN) techniques. Specifically, the minimum secrecy rate (MSR) among multiple eavesdroppers is maximized by jointly optimizing AAV deployment, signal and AN precoders, and FA positions. In particular, the worst-case MSR is considered by taking the channel uncertainties due to the uncertainty about eavesdropping locations into account. To tackle the highly coupled optimization variables and the channel uncertainties in the formulated problem, an efficient and robust algorithm is proposed. Particularly, the uncertain regions of eavesdroppers, whose shapes can be arbitrary, are disposed by constructing convex hull. In addition, two movement modes of FAs are considered, namely, free movement mode and zonal movement mode, for which different optimization techniques are applied, respectively. Numerical results show that, the proposed FA schemes boost security by exploiting additional spatial DoF rather than transmit power, while AN provides remarkable gains under high transmit power. Furthermore, the synergy between FA and AN results in a secure advantage that exceeds the sum of their individual contributions, achieving a balance between security and reliability under limited resources.

Off-grid Channel Estimation for Orthogonal Delay-Doppler Division Multiplexing Using Grid Refinement and Adjustment

Orthogonal delay-Doppler (DD) division multiplexing (ODDM) has been recently proposed as a promising multicarrier modulation scheme to tackle Doppler spread in high-mobility environments. Accurate channel estimation is of paramount importance to guarantee reliable communication for the ODDM, especially when the delays and Dopplers of the propagation paths are off-grid. In this paper, we propose a novel grid refinement and adjustment-based sparse Bayesian inference (GRASBI) scheme for DD domain channel estimation. The GRASBI involves first formulating the channel estimation problem as a sparse signal recovery through the introduction of a virtual DD grid. Then, an iterative process is proposed that involves (i) sparse Bayesian learning to estimate the channel parameters and (ii) a novel grid refinement and adjustment process to adjust the virtual grid points. The grid adjustment in GRASBI relies on the maximum likelihood principle to attain the adjustment and utilizes refined grids that have much higher resolution than the virtual grid. Moreover, a low-complexity grid refinement and adjustment-based channel estimation scheme is proposed, that can provides a good tradeoff between the estimation accuracy and the complexity. Finally, numerical results are provided to demonstrate the accuracy and efficiency of the proposed channel estimation schemes.

Rethinking: Deep-learning-based Demodulation and Decoding

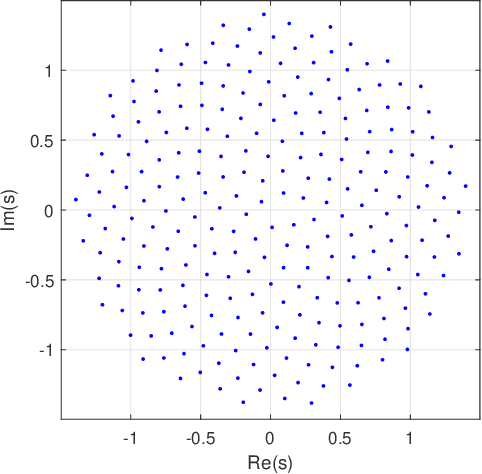
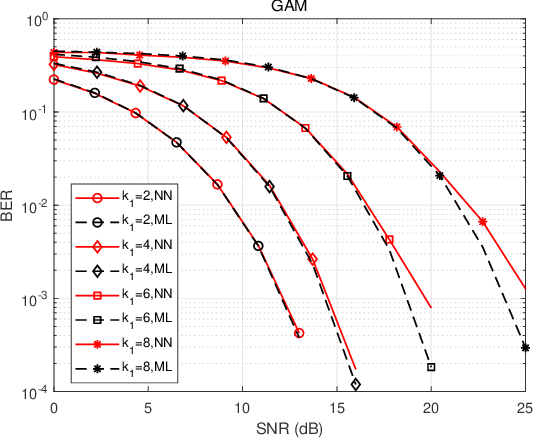
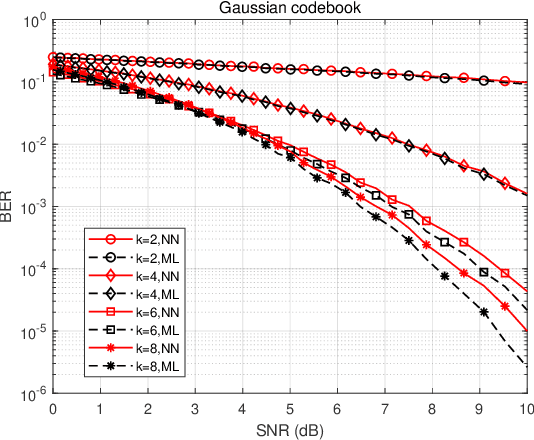
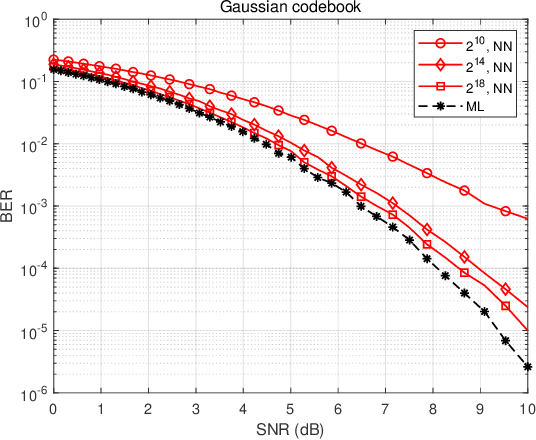
In this paper, we focus on the demodulation/decoding of the complex modulations/codes that approach the Shannon capacity. Theoretically, the maximum likelihood (ML) algorithm can achieve the optimal error performance whereas it has $\mathcal{O}(2^k)$ demodulation/decoding complexity with $k$ denoting the number of information bits. Recent progress in deep learning provides a new direction to tackle the demodulation and the decoding. The purpose of this paper is to analyze the feasibility of the neural network to demodulate/decode the complex modulations/codes close to the Shannon capacity and characterize the error performance and the complexity of the neural network. Regarding the neural network demodulator, we use the golden angle modulation (GAM), a promising modulation format that can offer the Shannon capacity approaching performance, to evaluate the demodulator. It is observed that the neural network demodulator can get a close performance to the ML-based method while it suffers from the lower complexity order in the low-order GAM. Regarding the neural network decoder, we use the Gaussian codebook, achieving the Shannon capacity, to evaluate the decoder. We also observe that the neural network decoder achieves the error performance close to the ML decoder with a much lower complexity order in the small Gaussian codebook. Limited by the current training resources, we cannot evaluate the performance of the high-order modulation and the long codeword. But, based on the results of the low-order GAM and the small Gaussian codebook, we boldly give our conjecture: the neural network demodulator/decoder is a strong candidate approach for demodulating/decoding the complex modulations/codes close to the Shannon capacity owing to the error performance of the near-ML algorithm and the lower complexity.

Low Complexity Kolmogorov-Smirnov Modulation Classification

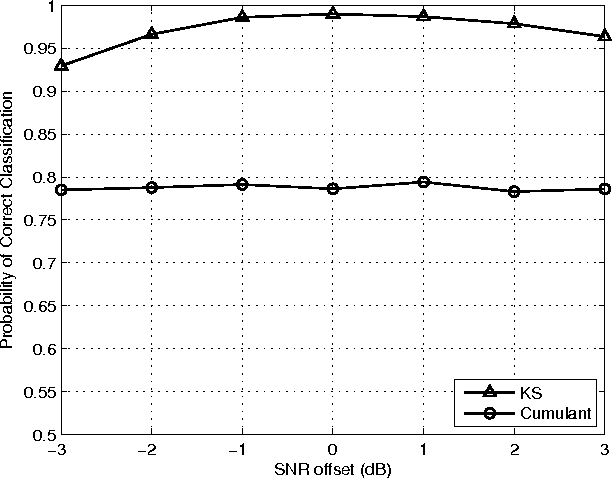
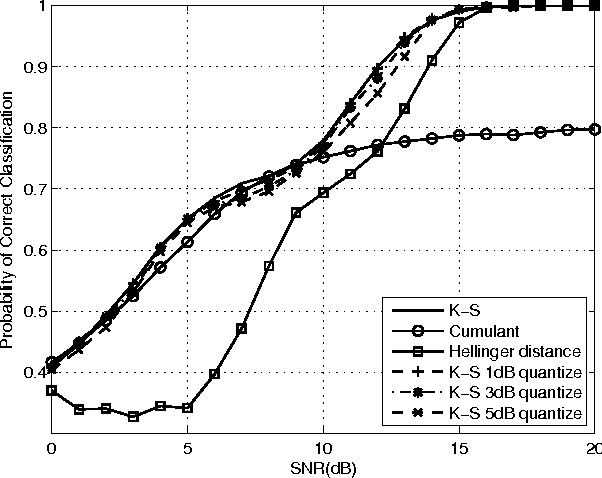
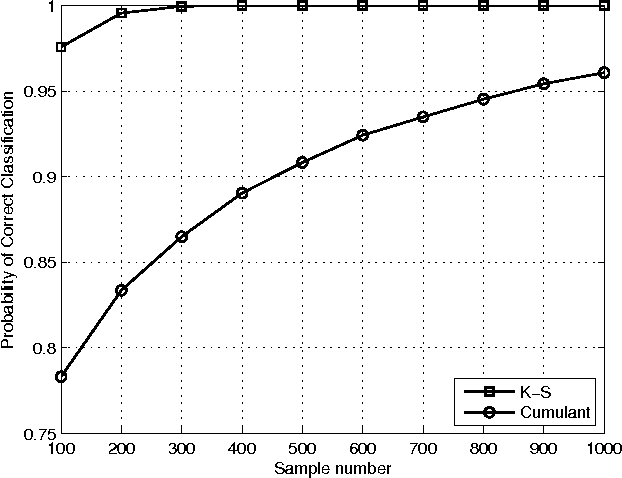
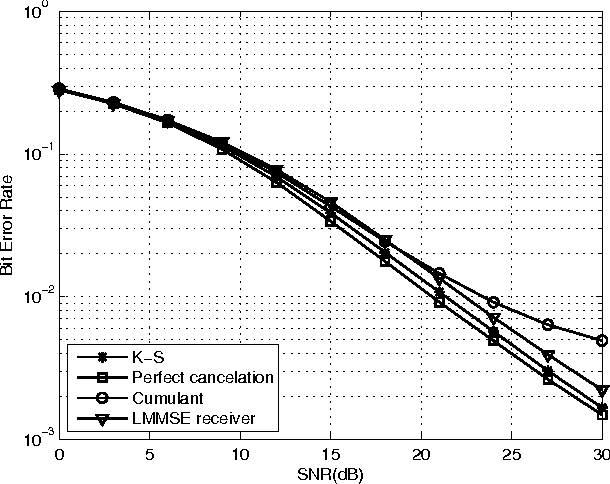
Kolmogorov-Smirnov (K-S) test-a non-parametric method to measure the goodness of fit, is applied for automatic modulation classification (AMC) in this paper. The basic procedure involves computing the empirical cumulative distribution function (ECDF) of some decision statistic derived from the received signal, and comparing it with the CDFs of the signal under each candidate modulation format. The K-S-based modulation classifier is first developed for AWGN channel, then it is applied to OFDM-SDMA systems to cancel multiuser interference. Regarding the complexity issue of K-S modulation classification, we propose a low-complexity method based on the robustness of the K-S classifier. Extensive simulation results demonstrate that compared with the traditional cumulant-based classifiers, the proposed K-S classifier offers superior classification performance and requires less number of signal samples (thus is fast).