Equivalence of Additive and Multiplicative Coupling in Spiking Neural Networks

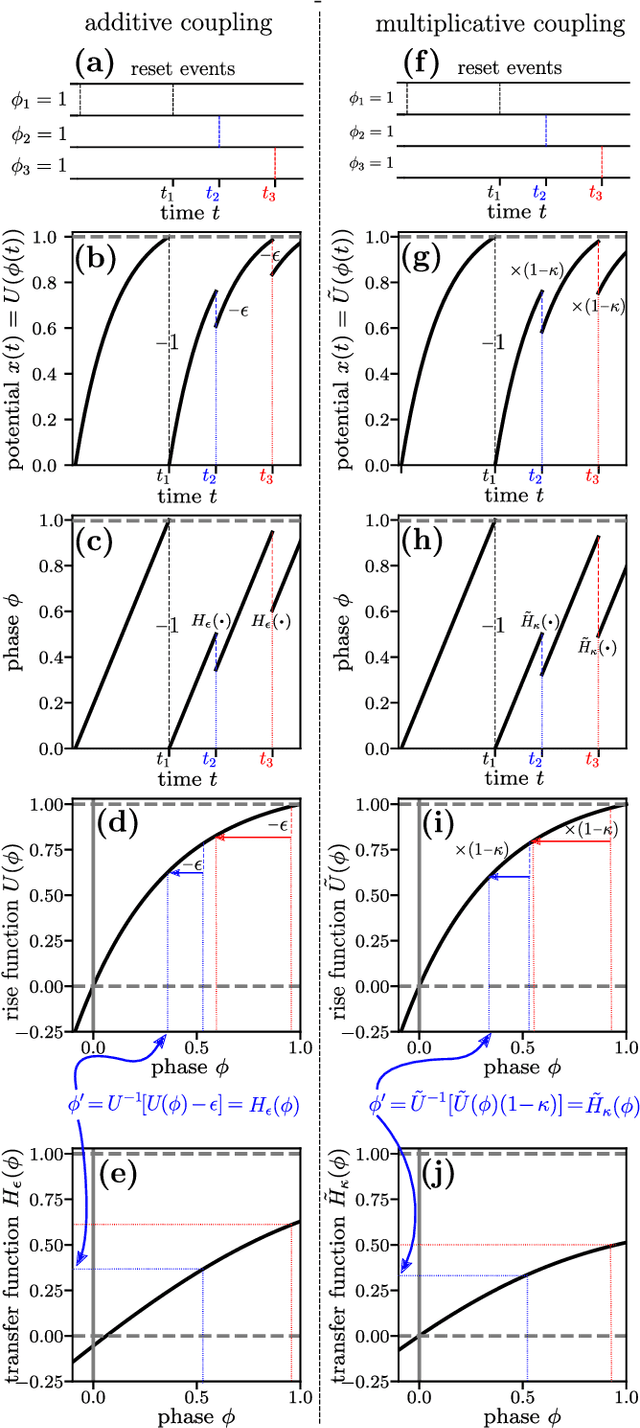
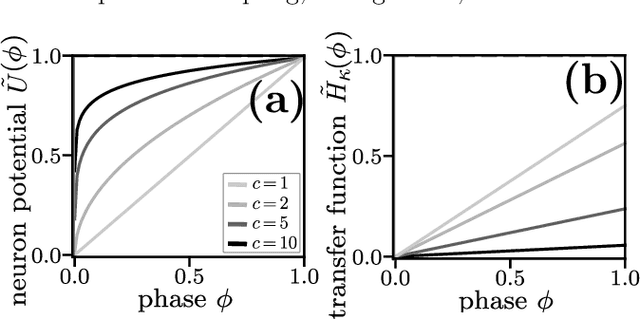
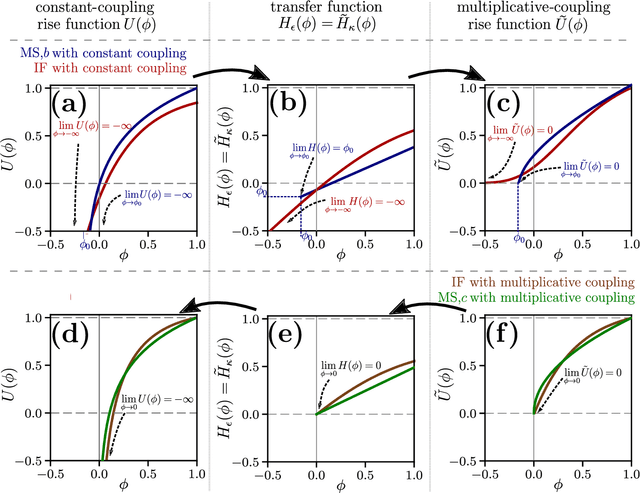
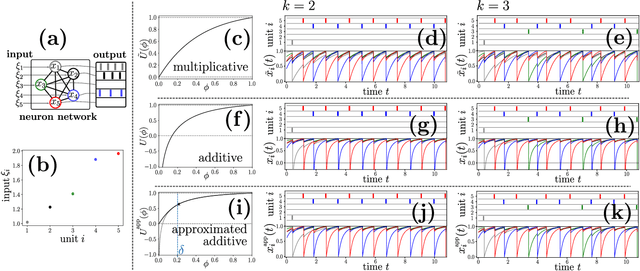
Spiking neural network models characterize the emergent collective dynamics of circuits of biological neurons and help engineer neuro-inspired solutions across fields. Most dynamical systems' models of spiking neural networks typically exhibit one of two major types of interactions: First, the response of a neuron's state variable to incoming pulse signals (spikes) may be additive and independent of its current state. Second, the response may depend on the current neuron's state and multiply a function of the state variable. Here we reveal that spiking neural network models with additive coupling are equivalent to models with multiplicative coupling for simultaneously modified intrinsic neuron time evolution. As a consequence, the same collective dynamics can be attained by state-dependent multiplicative and constant (state-independent) additive coupling. Such a mapping enables the transfer of theoretical insights between spiking neural network models with different types of interaction mechanisms as well as simpler and more effective engineering applications.

Stochastic facilitation in heteroclinic communication channels

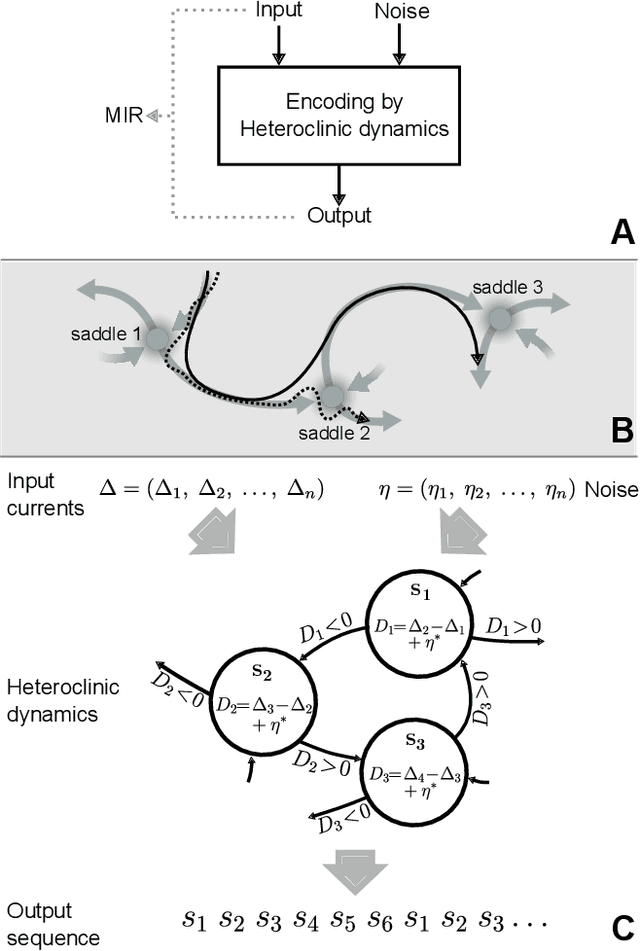
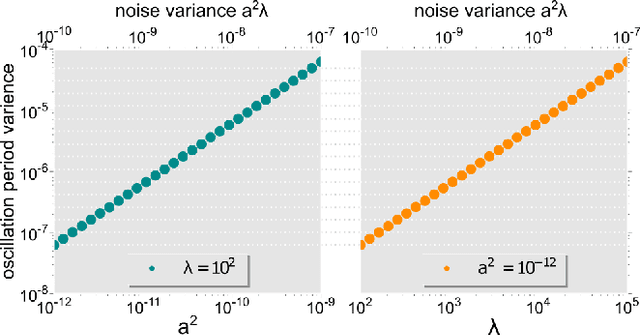
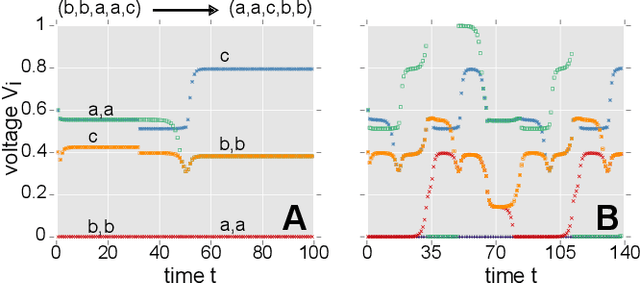
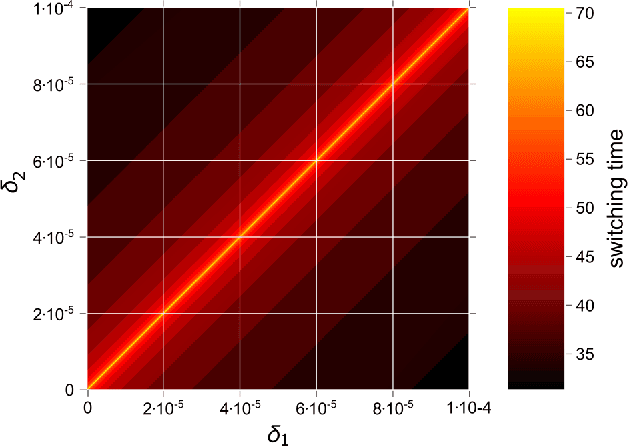
Biological neural systems encode and transmit information as patterns of activity tracing complex trajectories in high-dimensional state-spaces, inspiring alternative paradigms of information processing. Heteroclinic networks, naturally emerging in artificial neural systems, are networks of saddles in state-space that provide a transparent approach to generate complex trajectories via controlled switches among interconnected saddles. External signals induce specific switching sequences, thus dynamically encoding inputs as trajectories. Recent works have focused either on computational aspects of heteroclinic networks, i.e. Heteroclinic Computing, or their stochastic properties under noise. Yet, how well such systems may transmit information remains an open question. Here we investigate the information transmission properties of heteroclinic networks, studying them as communication channels. Choosing a tractable but representative system exhibiting a heteroclinic network, we investigate the mutual information rate (MIR) between input signals and the resulting sequences of states as the level of noise varies. Intriguingly, MIR does not decrease monotonically with increasing noise. Intermediate noise levels indeed maximize the information transmission capacity by promoting an increased yet controlled exploration of the underlying network of states. Complementing standard stochastic resonance, these results highlight the constructive effect of stochastic facilitation (i.e. noise-enhanced information transfer) on heteroclinic communication channels and possibly on more general dynamical systems exhibiting complex trajectories in state-space.

Controlled Perturbation-Induced Switching in Pulse-Coupled Oscillator Networks

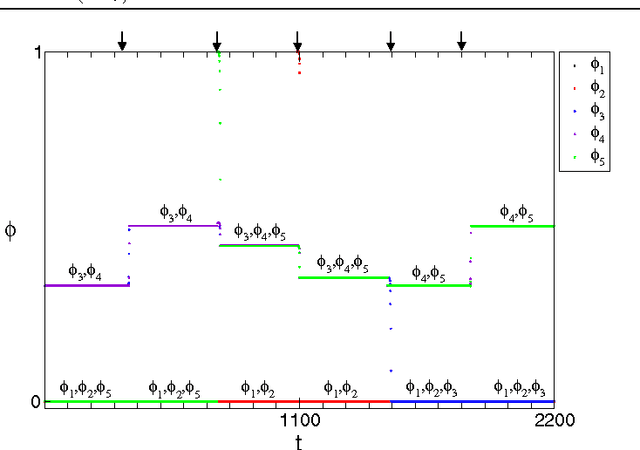
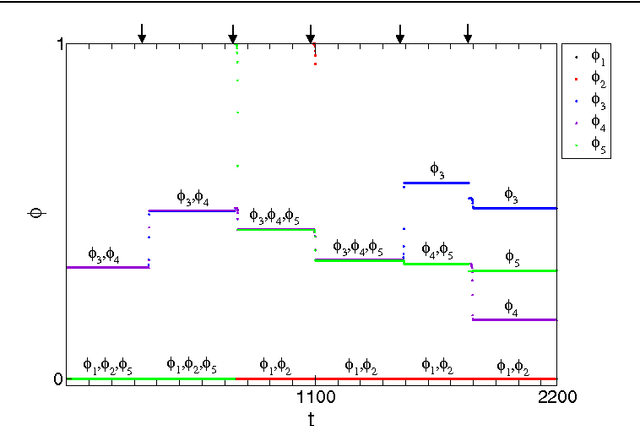
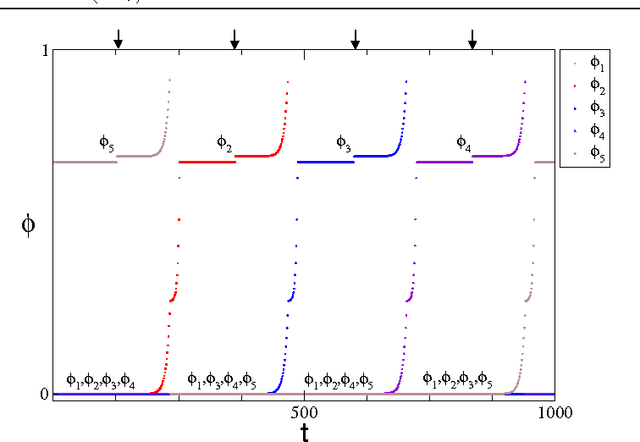
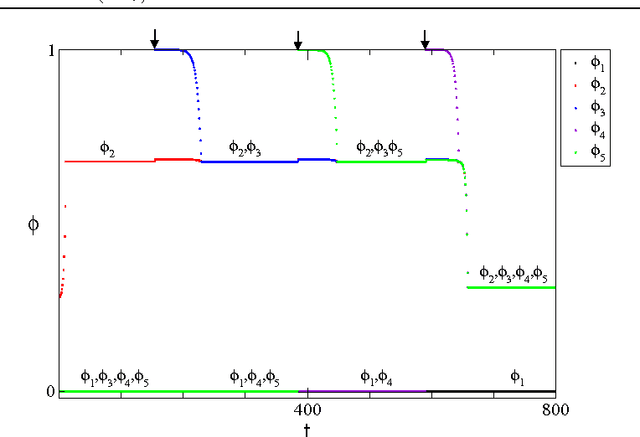
Pulse-coupled systems such as spiking neural networks exhibit nontrivial invariant sets in the form of attracting yet unstable saddle periodic orbits where units are synchronized into groups. Heteroclinic connections between such orbits may in principle support switching processes in those networks and enable novel kinds of neural computations. For small networks of coupled oscillators we here investigate under which conditions and how system symmetry enforces or forbids certain switching transitions that may be induced by perturbations. For networks of five oscillators we derive explicit transition rules that for two cluster symmetries deviate from those known from oscillators coupled continuously in time. A third symmetry yields heteroclinic networks that consist of sets of all unstable attractors with that symmetry and the connections between them. Our results indicate that pulse-coupled systems can reliably generate well-defined sets of complex spatiotemporal patterns that conform to specific transition rules. We briefly discuss possible implications for computation with spiking neural systems.