Tensor Laplacian Regularized Low-Rank Representation for Non-uniformly Distributed Data Subspace Clustering

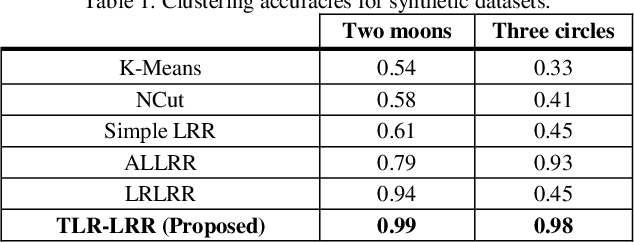
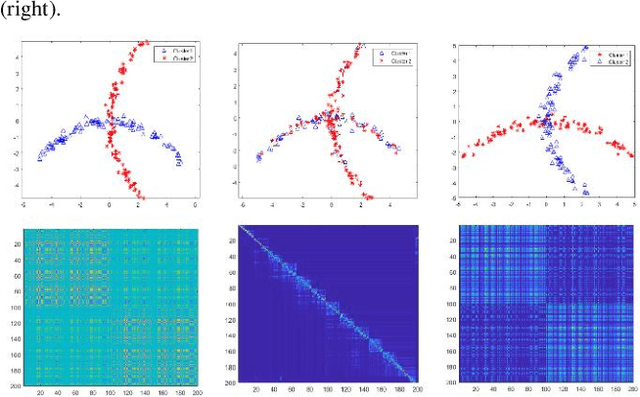
Low-Rank Representation (LRR) highly suffers from discarding the locality information of data points in subspace clustering, as it may not incorporate the data structure nonlinearity and the non-uniform distribution of observations over the ambient space. Thus, the information of the observational density is lost by the state-of-art LRR models, as they take a constant number of adjacent neighbors into account. This, as a result, degrades the subspace clustering accuracy in such situations. To cope with deficiency, in this paper, we propose to consider a hypergraph model to facilitate having a variable number of adjacent nodes and incorporating the locality information of the data. The sparsity of the number of subspaces is also taken into account. To do so, an optimization problem is defined based on a set of regularization terms and is solved by developing a tensor Laplacian-based algorithm. Extensive experiments on artificial and real datasets demonstrate the higher accuracy and precision of the proposed method in subspace clustering compared to the state-of-the-art methods. The outperformance of this method is more revealed in presence of inherent structure of the data such as nonlinearity, geometrical overlapping, and outliers.

Low-Rank Isomap Algorithm

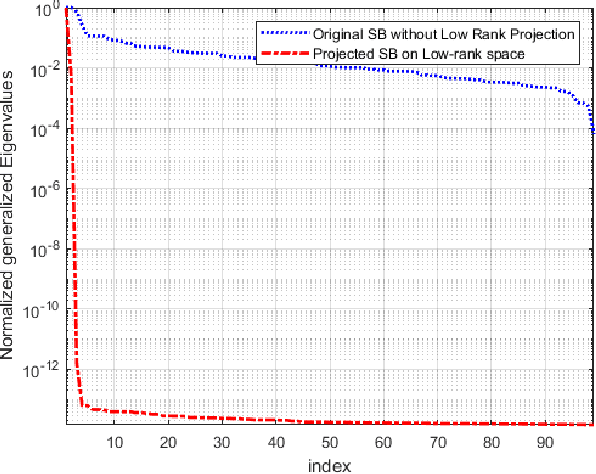
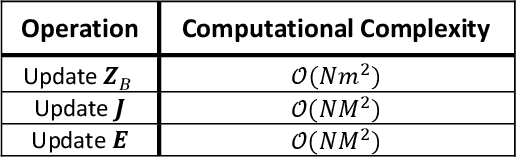
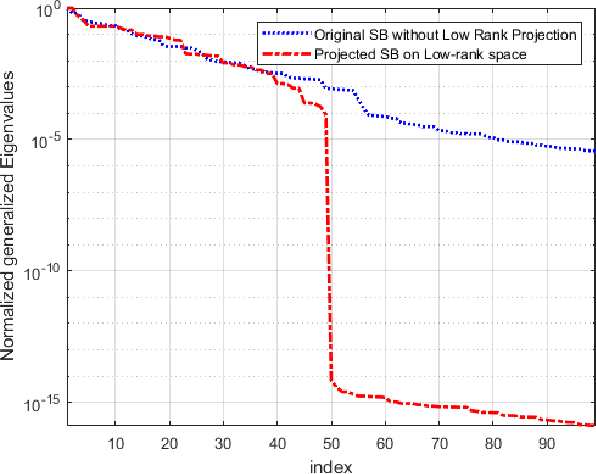
The Isomap is a well-known nonlinear dimensionality reduction method that highly suffers from computational complexity. Its computational complexity mainly arises from two stages; a) embedding a full graph on the data in the ambient space, and b) a complete eigenvalue decomposition. Although the reduction of the computational complexity of the graphing stage has been investigated, yet the eigenvalue decomposition stage remains a bottleneck in the problem. In this paper, we propose the Low-Rank Isomap algorithm by introducing a projection operator on the embedded graph from the ambient space to a low-rank latent space to facilitate applying the partial eigenvalue decomposition. This approach leads to reducing the complexity of Isomap to a linear order while preserving the structural information during the dimensionality reduction process. The superiority of the Low-Rank Isomap algorithm compared to some state-of-art algorithms is experimentally verified on facial image clustering in terms of speed and accuracy.