The Imandra Automated Reasoning System (system description)

We describe Imandra, a modern computational logic theorem prover designed to bridge the gap between decision procedures such as SMT, semi-automatic inductive provers of the Boyer-Moore family like ACL2, and interactive proof assistants for typed higher-order logics. Imandra's logic is computational, based on a pure subset of OCaml in which all functions are terminating, with restrictions on types and higher-order functions that allow conjectures to be translated into multi-sorted first-order logic with theories, including arithmetic and datatypes. Imandra has novel features supporting large-scale industrial applications, including a seamless integration of bounded and unbounded verification, first-class computable counterexamples, efficiently executable models and a cloud-native architecture supporting live multiuser collaboration. The core reasoning mechanisms of Imandra are (i) a semi-complete procedure for finding models of formulas in the logic mentioned above, centered around the lazy expansion of recursive functions, and (ii) an inductive waterfall and simplifier which "lifts" many Boyer-Moore ideas to our typed higher-order setting. These mechanisms are tightly integrated and subject to many forms of user control. Imandra's user interfaces include an interactive toplevel, Jupyter notebooks and asynchronous document-based verification (in the spirit of Isabelle's Prover IDE) with VS Code.

The Search for Computational Intelligence

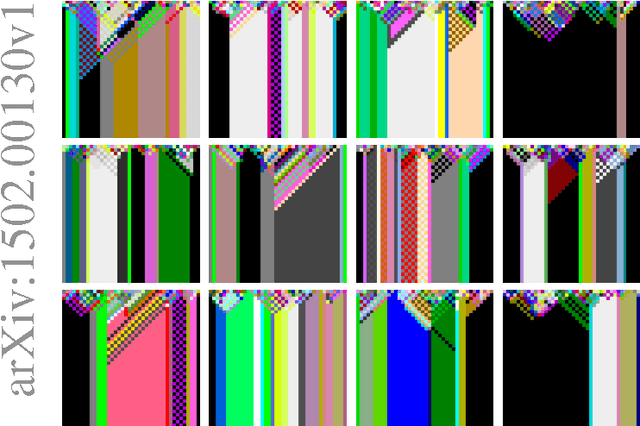
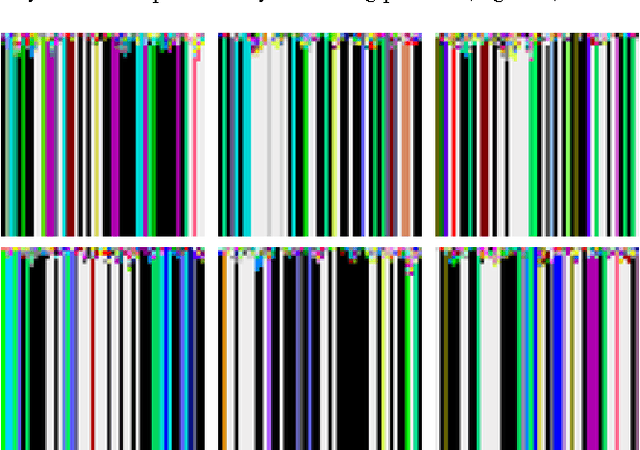
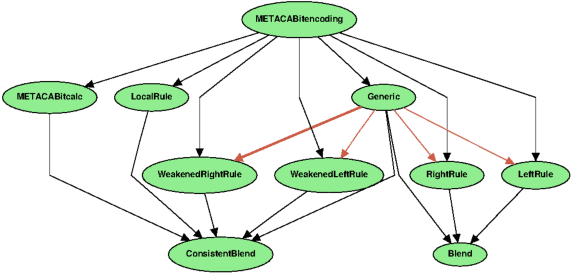
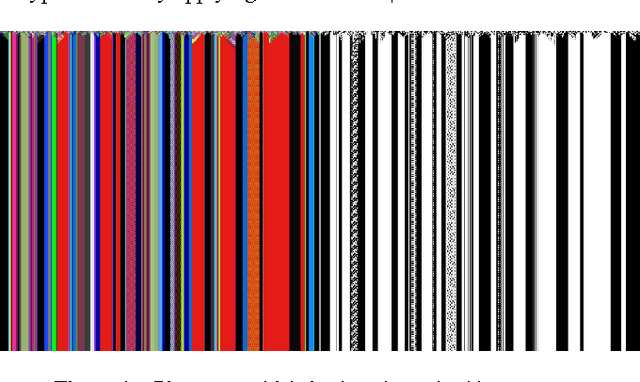
We define and explore in simulation several rules for the local evolution of generative rules for 1D and 2D cellular automata. Our implementation uses strategies from conceptual blending. We discuss potential applications to modelling social dynamics.

Towards Automated Proof Strategy Generalisation

The ability to automatically generalise (interactive) proofs and use such generalisations to discharge related conjectures is a very hard problem which remains unsolved. Here, we develop a notion of goal types to capture key properties of goals, which enables abstractions over the specific order and number of sub-goals arising when composing tactics. We show that the goal types form a lattice, and utilise this property in the techniques we develop to automatically generalise proof strategies in order to reuse it for proofs of related conjectures. We illustrate our approach with an example.