Cognitive Argumentation and the Suppression Task

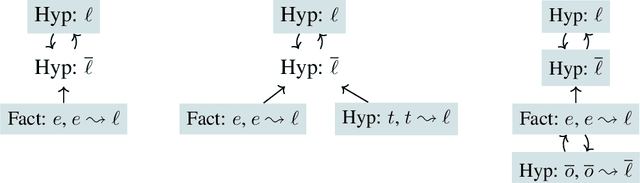
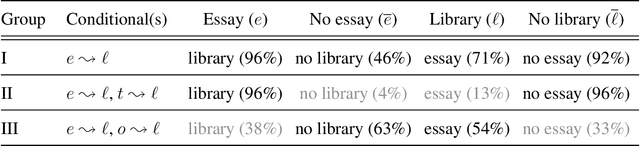
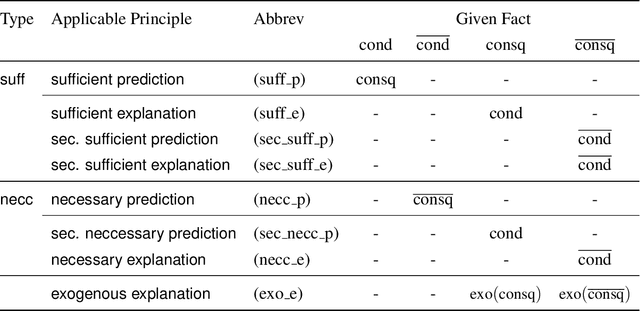
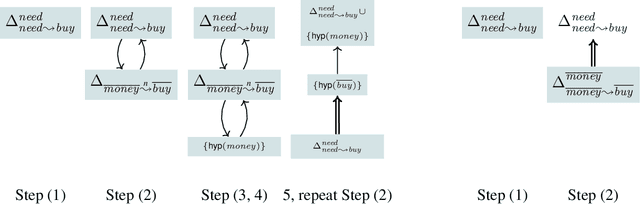
This paper addresses the challenge of modeling human reasoning, within a new framework called Cognitive Argumentation. This framework rests on the assumption that human logical reasoning is inherently a process of dialectic argumentation and aims to develop a cognitive model for human reasoning that is computational and implementable. To give logical reasoning a human cognitive form the framework relies on cognitive principles, based on empirical and theoretical work in Cognitive Science, to suitably adapt a general and abstract framework of computational argumentation from AI. The approach of Cognitive Argumentation is evaluated with respect to Byrne's suppression task, where the aim is not only to capture the suppression effect between different groups of people but also to account for the variation of reasoning within each group. Two main cognitive principles are particularly important to capture human conditional reasoning that explain the participants' responses: (i) the interpretation of a condition within a conditional as sufficient and/or necessary and (ii) the mode of reasoning either as predictive or explanatory. We argue that Cognitive Argumentation provides a coherent and cognitively adequate model for human conditional reasoning that allows a natural distinction between definite and plausible conclusions, exhibiting the important characteristics of context-sensitive and defeasible reasoning.

On the Relation between Weak Completion Semantics and Answer Set Semantics

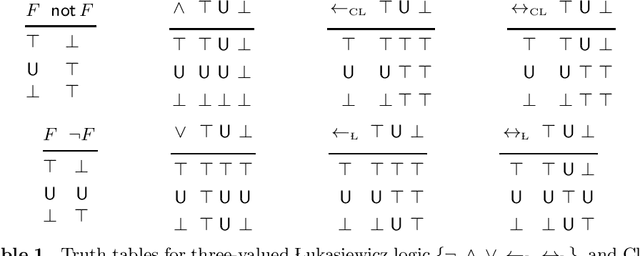
The Weak Completion Semantics (WCS) is a computational cognitive theory that has shown to be successful in modeling episodes of human reasoning. As the WCS is a recently developed logic programming approach, this paper investigates the correspondence of the WCS with respect to the well-established Answer Set Semantics (ASP). The underlying three-valued logic of both semantics is different and their models are evaluated with respect to different program transformations. We first illustrate these differences by the formal representation of some examples of a well-known psychological experiment, the suppression task. After that, we will provide a translation from logic programs understood under the WCS into logic programs understood under the ASP. In particular, we will show that logic programs under the WCS can be represented as logic programs under the ASP by means of a definition completion, where all defined atoms in a program must be false when their definitions are false.