Online Curvature-Aware Replay: Leveraging $\mathbf{2^{nd}}$ Order Information for Online Continual Learning

Online Continual Learning (OCL) models continuously adapt to nonstationary data streams, usually without task information. These settings are complex and many traditional CL methods fail, while online methods (mainly replay-based) suffer from instabilities after the task shift. To address this issue, we formalize replay-based OCL as a second-order online joint optimization with explicit KL-divergence constraints on replay data. We propose Online Curvature-Aware Replay (OCAR) to solve the problem: a method that leverages second-order information of the loss using a K-FAC approximation of the Fisher Information Matrix (FIM) to precondition the gradient. The FIM acts as a stabilizer to prevent forgetting while also accelerating the optimization in non-interfering directions. We show how to adapt the estimation of the FIM to a continual setting stabilizing second-order optimization for non-iid data, uncovering the role of the Tikhonov regularization in the stability-plasticity tradeoff. Empirical results show that OCAR outperforms state-of-the-art methods in continual metrics achieving higher average accuracy throughout the training process in three different benchmarks.

GAS-Norm: Score-Driven Adaptive Normalization for Non-Stationary Time Series Forecasting in Deep Learning

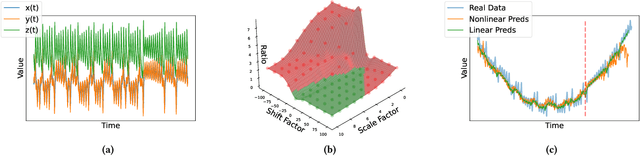
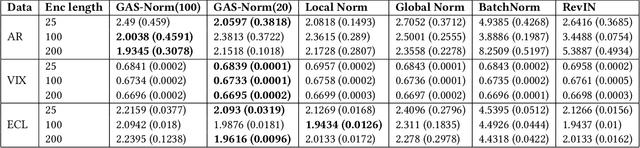
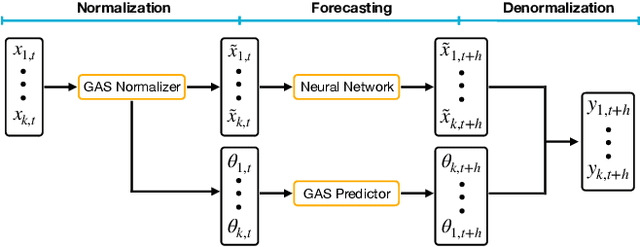
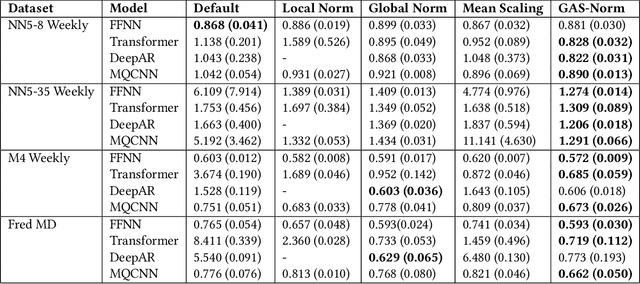
Despite their popularity, deep neural networks (DNNs) applied to time series forecasting often fail to beat simpler statistical models. One of the main causes of this suboptimal performance is the data non-stationarity present in many processes. In particular, changes in the mean and variance of the input data can disrupt the predictive capability of a DNN. In this paper, we first show how DNN forecasting models fail in simple non-stationary settings. We then introduce GAS-Norm, a novel methodology for adaptive time series normalization and forecasting based on the combination of a Generalized Autoregressive Score (GAS) model and a Deep Neural Network. The GAS approach encompasses a score-driven family of models that estimate the mean and variance at each new observation, providing updated statistics to normalize the input data of the deep model. The output of the DNN is eventually denormalized using the statistics forecasted by the GAS model, resulting in a hybrid approach that leverages the strengths of both statistical modeling and deep learning. The adaptive normalization improves the performance of the model in non-stationary settings. The proposed approach is model-agnostic and can be applied to any DNN forecasting model. To empirically validate our proposal, we first compare GAS-Norm with other state-of-the-art normalization methods. We then combine it with state-of-the-art DNN forecasting models and test them on real-world datasets from the Monash open-access forecasting repository. Results show that deep forecasting models improve their performance in 21 out of 25 settings when combined with GAS-Norm compared to other normalization methods.