Analyzing Downlink Coverage in Clustered Low Earth Orbit Satellite Constellations: A Stochastic Geometry Approach

Satellite networks are emerging as vital solutions for global connectivity beyond 5G. As companies such as SpaceX, OneWeb, and Amazon are poised to launch a large number of satellites in low Earth orbit, the heightened inter-satellite interference caused by mega-constellations has become a significant concern. To address this challenge, recent works have introduced the concept of satellite cluster networks where multiple satellites in a cluster collaborate to enhance the network performance. In order to investigate the performance of these networks, we propose mathematical analyses by modeling the locations of satellites and users using Poisson point processes, building on the success of stochastic geometry-based analyses for satellite networks. In particular, we suggest the lower and upper bounds of the coverage probability as functions of the system parameters, including satellite density, satellite altitude, satellite cluster area, path loss exponent, and Nakagami parameter $m$. We validate the analytical expressions by comparing them with simulation results. Our analyses can be used to design reliable satellite cluster networks by effectively estimating the impact of system parameters on the coverage performance.

Modeling and Analysis of GEO Satellite Networks

The extensive coverage offered by satellites makes them effective in enhancing service continuity for users on dynamic airborne and maritime platforms, such as airplanes and ships. In particular, geosynchronous Earth orbit (GEO) satellites ensure stable connectivity for terrestrial users due to their stationary characteristics when observed from Earth. This paper introduces a novel approach to model and analyze GEO satellite networks using stochastic geometry. We model the distribution of GEO satellites in the geostationary orbit according to a binomial point process (BPP) and examine satellite visibility depending on the terminal's latitude. Then, we identify potential distribution cases for GEO satellites and derive case probabilities based on the properties of the BPP. We also obtain the distance distributions between the terminal and GEO satellites and derive the coverage probability of the network. We further approximate the derived expressions using the Poisson limit theorem. Monte Carlo simulations are performed to validate the analytical findings, demonstrating a strong alignment between the analyses and simulations. The simplified analytical results can be used to estimate the coverage performance of GEO satellite networks by effectively modeling the positions of GEO satellites.

Satellite Clusters Flying in Formation: Orbital Configuration-Dependent Performance Analyses

This paper considers a downlink satellite communication system where a satellite cluster, i.e., a satellite swarm consisting of one leader and multiple follower satellites, serves a ground terminal. The satellites in the cluster form either a linear or circular formation moving in a group and cooperatively send their signals by maximum ratio transmission precoding. We first conduct a coordinate transformation to effectively capture the relative positions of satellites in the cluster. Next, we derive an exact expression for the orbital configuration-dependent outage probability under the Nakagami fading by using the distribution of the sum of independent Gamma random variables. In addition, we obtain a simpler approximated expression for the outage probability with the help of second-order moment-matching. We also analyze asymptotic behavior in the high signal-to-noise ratio regime and the diversity order of the outage performance. Finally, we verify the analytical results through Monte Carlo simulations. Our analytical results provide the performance of satellite cluster-based communication systems based on specific orbital configurations, which can be used to design reliable satellite clusters in terms of cluster size, formation, and orbits.

Performance Analysis of Satellite Communication System Under the Shadowed-Rician Fading: A Stochastic Geometry Approach

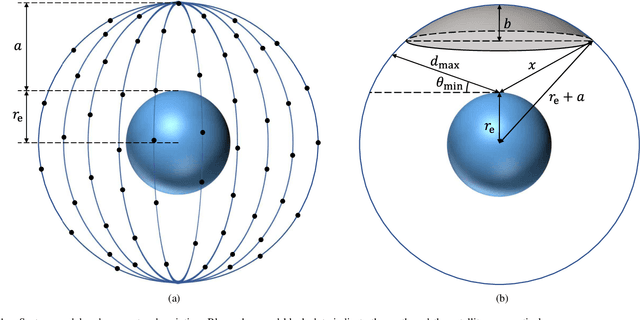
In this paper, we consider a downlink satellite communication system where multiple satellites are uniformly distributed over a sphere at a certain altitude. We analytically derive three things: 1) the satellite-visible probability for a given location, which is defined as the probability that a terminal sees at least one satellite above the minimum elevation angle, i.e., a pre-defined elevation angle above which the terminal can be served by a satellite, 2) the distribution of distance between the terminal and serving satellite when the terminal is associated with the nearest satellite, and 3) the exact expressions for the outage probability and throughput of the system. With the derived expressions, the system throughput maximization problem is formulated under the satellite-visibility and outage constraints. To solve the problem, we reformulate the problem with bounded feasible sets and obtain the optimal solution by using an exhaustive search. Using the Poisson limit theorem, we derive approximated expressions for the satellite-visible probability, outage probability, and system throughput, which reduce computational complexity of performance evaluation and search time for the optimal solution of the throughput maximization problem. Simulation results perfectly match the derived exact expressions for the outage probability and system throughput. It is also shown that the analytical results of the approximated expressions are fairly close to those of the exact expressions.