Distributed Nonparametric Estimation: from Sparse to Dense Samples per Terminal

Consider the communication-constrained problem of nonparametric function estimation, in which each distributed terminal holds multiple i.i.d. samples. Under certain regularity assumptions, we characterize the minimax optimal rates for all regimes, and identify phase transitions of the optimal rates as the samples per terminal vary from sparse to dense. This fully solves the problem left open by previous works, whose scopes are limited to regimes with either dense samples or a single sample per terminal. To achieve the optimal rates, we design a layered estimation protocol by exploiting protocols for the parametric density estimation problem. We show the optimality of the protocol using information-theoretic methods and strong data processing inequalities, and incorporating the classic balls and bins model. The optimal rates are immediate for various special cases such as density estimation, Gaussian, binary, Poisson and heteroskedastic regression models.

Adaptive Refinement Protocols for Distributed Distribution Estimation under $\ell^p$-Losses

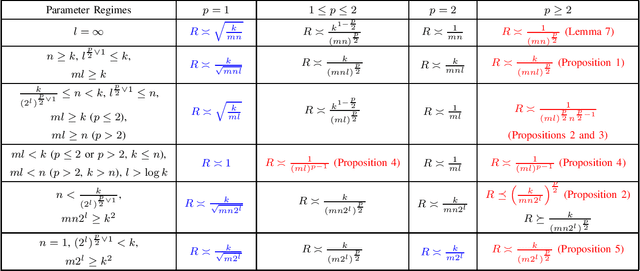
Consider the communication-constrained estimation of discrete distributions under $\ell^p$ losses, where each distributed terminal holds multiple independent samples and uses limited number of bits to describe the samples. We obtain the minimax optimal rates of the problem in most parameter regimes. An elbow effect of the optimal rates at $p=2$ is clearly identified. To show the optimal rates, we first design estimation protocols to achieve them. The key ingredient of these protocols is to introduce adaptive refinement mechanisms, which first generate rough estimate by partial information and then establish refined estimate in subsequent steps guided by the rough estimate. The protocols leverage successive refinement, sample compression and thresholding methods to achieve the optimal rates in different parameter regimes. The optimality of the protocols is shown by deriving compatible minimax lower bounds.