Efficient, Responsive, and Robust Hopping on Deformable Terrain

Legged robot locomotion is hindered by a mismatch between applications where legs can outperform wheels or treads, most of which feature deformable substrates, and existing tools for planning and control, most of which assume flat, rigid substrates. In this study we focus on the ramifications of plastic terrain deformation on the hop-to-hop energy dynamics of a spring-legged monopedal hopping robot animated by a switched-compliance energy injection controller. From this deliberately simple robot-terrain model, we derive a hop-to-hop energy return map, and we use physical experiments and simulations to validate the hop-to-hop energy map for a real robot hopping on a real deformable substrate. The dynamical properties (fixed points, eigenvalues, basins of attraction) of this map provide insights into efficient, responsive, and robust locomotion on deformable terrain. Specifically, we identify constant-fixed-point surfaces in a controller parameter space that suggest it is possible to tune control parameters for efficiency or responsiveness while targeting a desired gait energy level. We also identify conditions under which fixed points of the energy map are globally stable, and we further characterize the basins of attraction of fixed points when these conditions are not satisfied. We conclude by discussing the implications of this hop-to-hop energy map for planning, control, and estimation for efficient, agile, and robust legged locomotion on deformable terrain.

The Soft Landing Problem: Minimizing Energy Loss by a Legged Robot Impacting Yielding Terrain

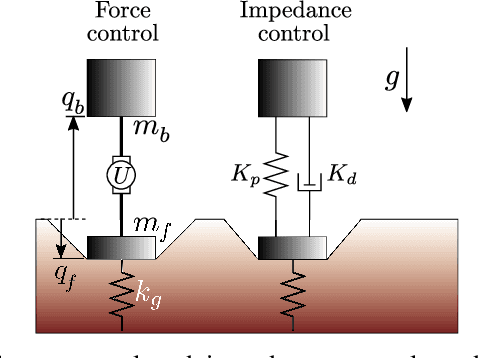
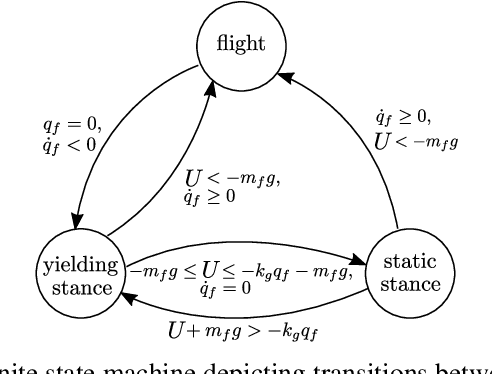
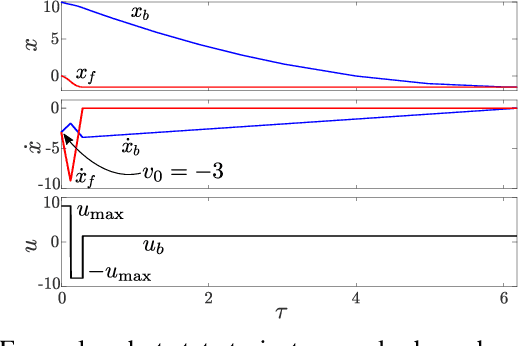
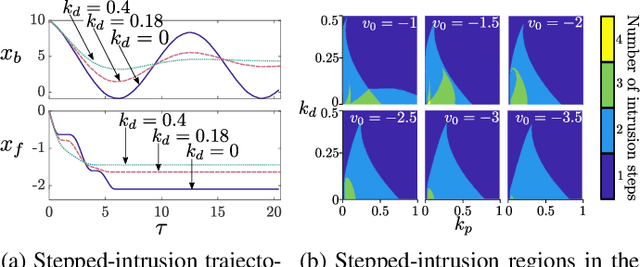
Enabling robots to walk and run on yielding terrain is increasingly vital to endeavors ranging from disaster response to extraterrestrial exploration. While dynamic legged locomotion on rigid ground is challenging enough, yielding terrain presents additional challenges such as permanent ground deformation which dissipates energy. In this paper, we examine the soft landing problem: given some impact momentum, bring the robot to rest while minimizing foot penetration depth. To gain insight into properties of penetration depth-minimizing control policies, we formulate a constrained optimal control problem and obtain a bang-bang open-loop force profile. Motivated by examples from biology and recent advances in legged robotics, we also examine impedance-control solutions to the dimensionless soft landing problem. Through simulations, we find that optimal impedance reduces penetration depth nearly as much as the open-loop force profile, while remaining robust to model uncertainty. Through simulations and experiments, we find that the solution space is rich, exhibiting qualitatively different relationships between impact velocity and the optimal impedance for small and large dimensionless impact velocities. Lastly, we discuss the relevance of this work to minimum-cost-of-transport locomotion for several actuator design choices.