Chih-Min Huang
Variational Quantum Reinforcement Learning via Evolutionary Optimization
Sep 01, 2021
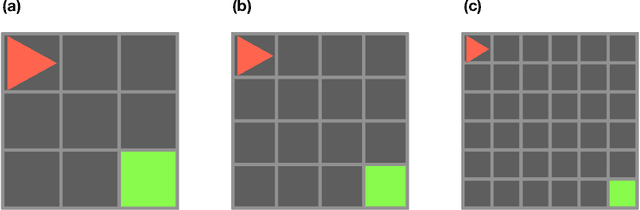
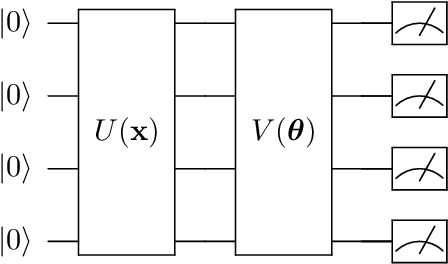
Abstract:Recent advance in classical reinforcement learning (RL) and quantum computation (QC) points to a promising direction of performing RL on a quantum computer. However, potential applications in quantum RL are limited by the number of qubits available in the modern quantum devices. Here we present two frameworks of deep quantum RL tasks using a gradient-free evolution optimization: First, we apply the amplitude encoding scheme to the Cart-Pole problem; Second, we propose a hybrid framework where the quantum RL agents are equipped with hybrid tensor network-variational quantum circuit (TN-VQC) architecture to handle inputs with dimensions exceeding the number of qubits. This allows us to perform quantum RL on the MiniGrid environment with 147-dimensional inputs. We demonstrate the quantum advantage of parameter saving using the amplitude encoding. The hybrid TN-VQC architecture provides a natural way to perform efficient compression of the input dimension, enabling further quantum RL applications on noisy intermediate-scale quantum devices.
An end-to-end trainable hybrid classical-quantum classifier
Feb 04, 2021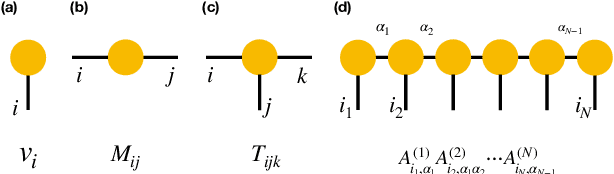
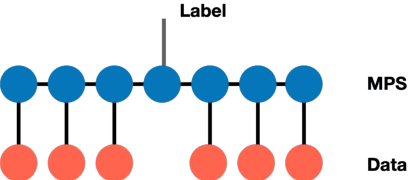
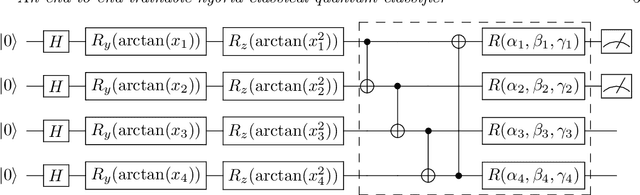
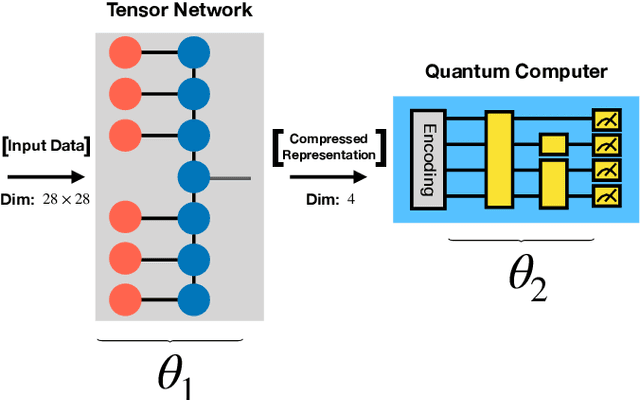
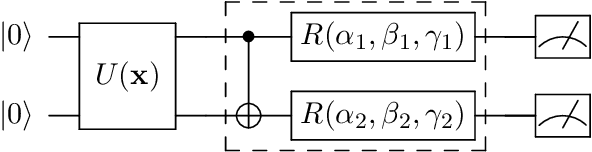
Abstract:We introduce a hybrid model combining a quantum-inspired tensor network and a variational quantum circuit to perform supervised learning tasks. This architecture allows for the classical and quantum parts of the model to be trained simultaneously, providing an end-to-end training framework. We show that compared to the principal component analysis, a tensor network based on the matrix product state with low bond dimensions performs better as a feature extractor for the input data of the variational quantum circuit in the binary and ternary classification of MNIST and Fashion-MNIST datasets. The architecture is highly adaptable and the classical-quantum boundary can be adjusted according the availability of the quantum resource by exploiting the correspondence between tensor networks and quantum circuits.
Hybrid quantum-classical classifier based on tensor network and variational quantum circuit
Nov 30, 2020

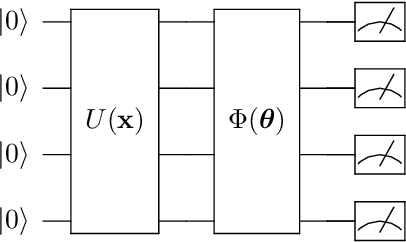

Abstract:One key step in performing quantum machine learning (QML) on noisy intermediate-scale quantum (NISQ) devices is the dimension reduction of the input data prior to their encoding. Traditional principle component analysis (PCA) and neural networks have been used to perform this task; however, the classical and quantum layers are usually trained separately. A framework that allows for a better integration of the two key components is thus highly desirable. Here we introduce a hybrid model combining the quantum-inspired tensor networks (TN) and the variational quantum circuits (VQC) to perform supervised learning tasks, which allows for an end-to-end training. We show that a matrix product state based TN with low bond dimensions performs better than PCA as a feature extractor to compress data for the input of VQCs in the binary classification of MNIST dataset. The architecture is highly adaptable and can easily incorporate extra quantum resource when available.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge