Benyang Gong
A Covariance Adaptive Student's t Based Kalman Filter
Sep 18, 2023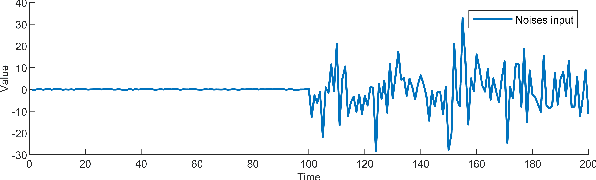
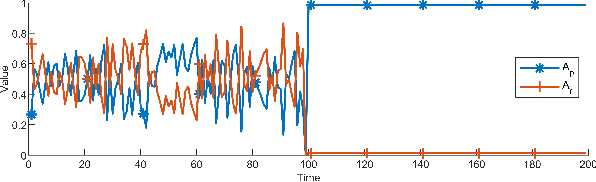
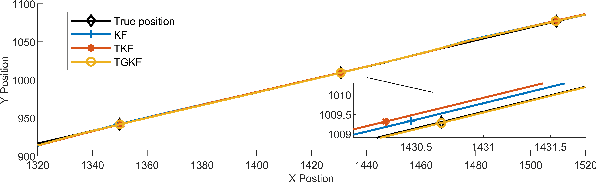
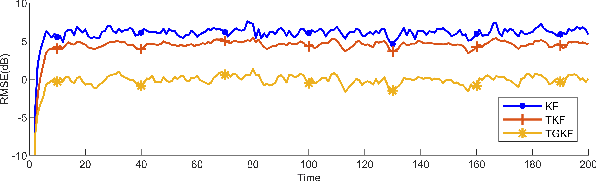
Abstract:In the classical Kalman filter(KF), the estimated state is a linear combination of the one-step predicted state and measurement state, their confidence level change when the prediction mean square error matrix and covariance matrix of measurement noise vary. The existing student's t based Kalman filter(TKF) works similarly to the way KF works, they both work well with impulse noise, but when it comes to Gaussian noise, TKF encounters an adjustment limit of the confidence level, this can lead to inaccuracies in such situations. This brief optimizes TKF by using the Gaussian mixture model(GMM), which generates a reasonable covariance matrix from the measurement noise to replace the one used in the existing algorithm and breaks the adjustment limit of the confidence level. At the end of the brief, the performance of the covariance adaptive student's t based Kalman filter(TGKF) is verified.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge