PROPm Allocations of Indivisible Goods to Multiple Agents

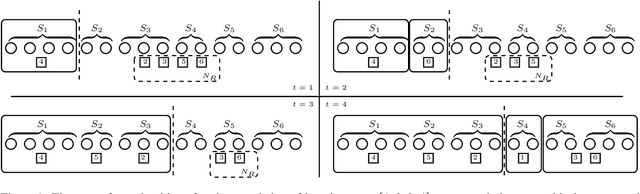
We study the classic problem of fairly allocating a set of indivisible goods among a group of agents, and focus on the notion of approximate proportionality known as PROPm. Prior work showed that there exists an allocation that satisfies this notion of fairness for instances involving up to five agents, but fell short of proving that this is true in general. We extend this result to show that a PROPm allocation is guaranteed to exist for all instances, independent of the number of agents or goods. Our proof is constructive, providing an algorithm that computes such an allocation and, unlike prior work, the running time of this algorithm is polynomial in both the number of agents and the number of goods.

Detecting corruption in single-bidder auctions via positive-unlabelled learning

In research and policy-making guidelines, the single-bidder rate is a commonly used proxy of corruption in public procurement used but ipso facto this is not evidence of a corrupt auction, but an uncompetitive auction. And while an uncompetitive auction could arise due to a corrupt procurer attempting to conceal the transaction, but it could also be a result of geographic isolation, monopolist presence, or other structural factors. In this paper we use positive-unlabelled classification to attempt to separate public procurement auctions in the Russian Federation into auctions that are probably fair, and those that are suspicious.

Achieving Proportionality up to the Maximin Item with Indivisible Goods

We study the problem of fairly allocating indivisible goods and focus on the classic fairness notion of proportionality. The indivisibility of the goods is long known to pose highly non-trivial obstacles to achieving fairness, and a very vibrant line of research has aimed to circumvent them using appropriate notions of approximate fairness. Recent work has established that even approximate versions of proportionality (PROPx) may be impossible to achieve even for small instances, while the best known achievable approximations (PROP1) are much weaker. We introduce the notion of proportionality up to the maximin item (PROPm) and show how to reach an allocation satisfying this notion for any instance involving up to five agents with additive valuations. PROPm provides a well motivated middle-ground between PROP1 and PROPx, while also capturing some elements of the well-studied maximin share (MMS) benchmark: another relaxation of proportionality that has attracted a lot of attention.