Towards Complete Causal Explanation with Expert Knowledge

We study the problem of restricting Markov equivalence classes of maximal ancestral graphs (MAGs) containing certain edge marks, which we refer to as expert knowledge. MAGs forming a Markov equivalence class can be uniquely represented by an essential ancestral graph. We seek to learn the restriction of the essential ancestral graph containing the proposed expert knowledge. Our contributions are several-fold. First, we prove certain properties for the entire Markov equivalence class including a conjecture from Ali et al. (2009). Second, we present three sound graphical orientation rules, two of which generalize previously known rules, for adding expert knowledge to an essential graph. We also show that some orientation rules of Zhang (2008) are not needed for restricting the Markov equivalence class with expert knowledge. We provide an algorithm for including this expert knowledge and show that our algorithm is complete in certain settings i.e., in these settings, the output of our algorithm is a restricted essential ancestral graph. We conjecture this algorithm is complete generally. Outside of our specified settings, we provide an algorithm for checking whether a graph is a restricted essential graph and discuss its runtime. This work can be seen as a generalization of Meek (1995).

Robustly estimating heterogeneity in factorial data using Rashomon Partitions

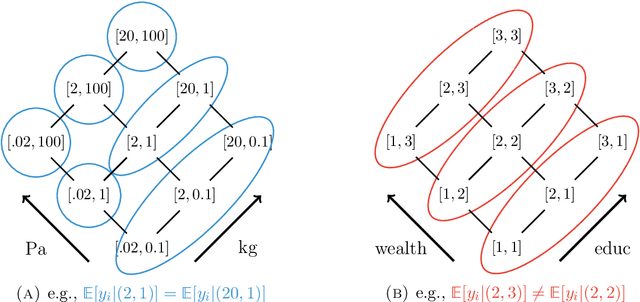
Many statistical analyses, in both observational data and randomized control trials, ask: how does the outcome of interest vary with combinations of observable covariates? How do various drug combinations affect health outcomes, or how does technology adoption depend on incentives and demographics? Our goal is to partition this factorial space into ``pools'' of covariate combinations where the outcome differs across the pools (but not within a pool). Existing approaches (i) search for a single ``optimal'' partition under assumptions about the association between covariates or (ii) sample from the entire set of possible partitions. Both these approaches ignore the reality that, especially with correlation structure in covariates, many ways to partition the covariate space may be statistically indistinguishable, despite very different implications for policy or science. We develop an alternative perspective, called Rashomon Partition Sets (RPSs). Each item in the RPS partitions the space of covariates using a tree-like geometry. RPSs incorporate all partitions that have posterior values near the maximum a posteriori partition, even if they offer substantively different explanations, and do so using a prior that makes no assumptions about associations between covariates. This prior is the $\ell_0$ prior, which we show is minimax optimal. Given the RPS we calculate the posterior of any measurable function of the feature effects vector on outcomes, conditional on being in the RPS. We also characterize approximation error relative to the entire posterior and provide bounds on the size of the RPS. Simulations demonstrate this framework allows for robust conclusions relative to conventional regularization techniques. We apply our method to three empirical settings: price effects on charitable giving, chromosomal structure (telomere length), and the introduction of microfinance.