Anna Schuhmann
Metric Dynamic Equilibrium Logic
Jan 19, 2024Abstract:In temporal extensions of Answer Set Programming (ASP) based on linear-time, the behavior of dynamic systems is captured by sequences of states. While this representation reflects their relative order, it abstracts away the specific times associated with each state. In many applications, however, timing constraints are important like, for instance, when planning and scheduling go hand in hand. We address this by developing a metric extension of linear-time Dynamic Equilibrium Logic, in which dynamic operators are constrained by intervals over integers. The resulting Metric Dynamic Equilibrium Logic provides the foundation of an ASP-based approach for specifying qualitative and quantitative dynamic constraints. As such, it constitutes the most general among a whole spectrum of temporal extensions of Equilibrium Logic. In detail, we show that it encompasses Temporal, Dynamic, Metric, and regular Equilibrium Logic, as well as its classic counterparts once the law of the excluded middle is added.
Metric Temporal Equilibrium Logic over Timed Traces
Apr 28, 2023Abstract:In temporal extensions of Answer Set Programming (ASP) based on linear-time, the behavior of dynamic systems is captured by sequences of states. While this representation reflects their relative order, it abstracts away the specific times associated with each state. However, timing constraints are important in many applications like, for instance, when planning and scheduling go hand in hand. We address this by developing a metric extension of linear-time temporal equilibrium logic, in which temporal operators are constrained by intervals over natural numbers. The resulting Metric Equilibrium Logic provides the foundation of an ASP-based approach for specifying qualitative and quantitative dynamic constraints. To this end, we define a translation of metric formulas into monadic first-order formulas and give a correspondence between their models in Metric Equilibrium Logic and Monadic Quantified Equilibrium Logic, respectively. Interestingly, our translation provides a blue print for implementation in terms of ASP modulo difference constraints.
Temporal Answer Set Programming
Sep 14, 2020



Abstract:We present an overview on Temporal Logic Programming under the perspective of its application for Knowledge Representation and declarative problem solving. Such programs are the result of combining usual rules with temporal modal operators, as in Linear-time Temporal Logic (LTL). We focus on recent results of the non-monotonic formalism called Temporal Equilibrium Logic (TEL) that is defined for the full syntax of LTL, but performs a model selection criterion based on Equilibrium Logic, a well known logical characterization of Answer Set Programming (ASP). We obtain a proper extension of the stable models semantics for the general case of arbitrary temporal formulas. We recall the basic definitions for TEL and its monotonic basis, the temporal logic of Here-and-There (THT), and study the differences between infinite and finite traces. We also provide other useful results, such as the translation into other formalisms like Quantified Equilibrium Logic or Second-order LTL, and some techniques for computing temporal stable models based on automata. In a second part, we focus on practical aspects, defining a syntactic fragment called temporal logic programs closer to ASP, and explain how this has been exploited in the construction of the solver TELINGO.
Towards Metric Temporal Answer Set Programming
Aug 08, 2020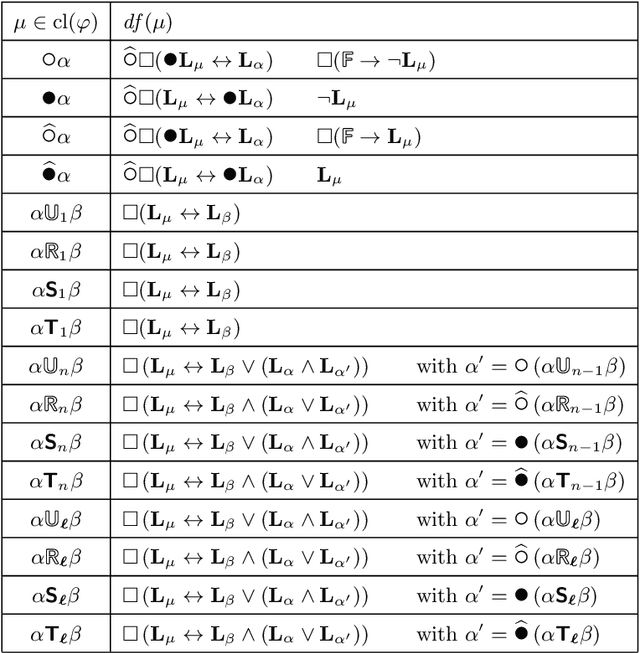
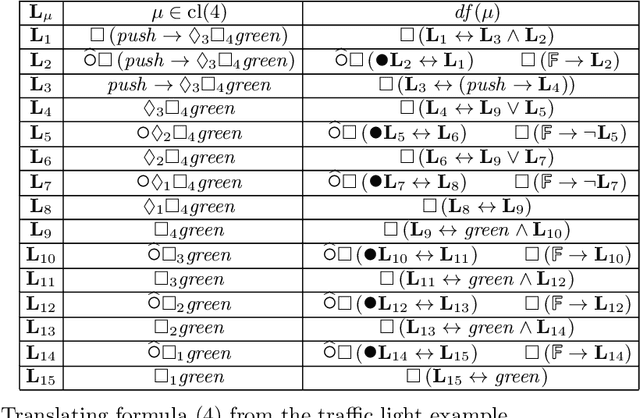
Abstract:We elaborate upon the theoretical foundations of a metric temporal extension of Answer Set Programming. In analogy to previous extensions of ASP with constructs from Linear Temporal and Dynamic Logic, we accomplish this in the setting of the logic of Here-and-There and its non-monotonic extension, called Equilibrium Logic. More precisely, we develop our logic on the same semantic underpinnings as its predecessors and thus use a simple time domain of bounded time steps. This allows us to compare all variants in a uniform framework and ultimately combine them in a common implementation.
Temporal Answer Set Programming on Finite Traces
Apr 26, 2018
Abstract:In this paper, we introduce an alternative approach to Temporal Answer Set Programming that relies on a variation of Temporal Equilibrium Logic (TEL) for finite traces. This approach allows us to even out the expressiveness of TEL over infinite traces with the computational capacity of (incremental) Answer Set Programming (ASP). Also, we argue that finite traces are more natural when reasoning about action and change. As a result, our approach is readily implementable via multi-shot ASP systems and benefits from an extension of ASP's full-fledged input language with temporal operators. This includes future as well as past operators whose combination offers a rich temporal modeling language. For computation, we identify the class of temporal logic programs and prove that it constitutes a normal form for our approach. Finally, we outline two implementations, a generic one and an extension of clingo.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge