Andrea De Domenico
A Meta-Learning Algorithm for Interrogative Agendas
Jan 04, 2023Abstract:Explainability is a key challenge and a major research theme in AI research for developing intelligent systems that are capable of working with humans more effectively. An obvious choice in developing explainable intelligent systems relies on employing knowledge representation formalisms which are inherently tailored towards expressing human knowledge e.g., interrogative agendas. In the scope of this work, we focus on formal concept analysis (FCA), a standard knowledge representation formalism, to express interrogative agendas, and in particular to categorize objects w.r.t. a given set of features. Several FCA-based algorithms have already been in use for standard machine learning tasks such as classification and outlier detection. These algorithms use a single concept lattice for such a task, meaning that the set of features used for the categorization is fixed. Different sets of features may have different importance in that categorization, we call a set of features an agenda. In many applications a correct or good agenda for categorization is not known beforehand. In this paper, we propose a meta-learning algorithm to construct a good interrogative agenda explaining the data. Such algorithm is meant to call existing FCA-based classification and outlier detection algorithms iteratively, to increase their accuracy and reduce their sample complexity. The proposed method assigns a measure of importance to different set of features used in the categorization, hence making the results more explainable.
Complexity assessments for decidable fragments of Set Theory. III: A quadratic reduction of constraints over nested sets to Boolean formulae
Dec 09, 2021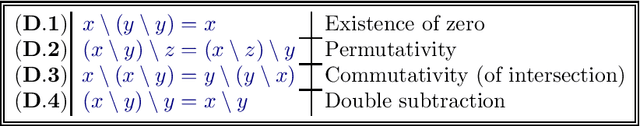
Abstract:As a contribution to quantitative set-theoretic inferencing, a translation is proposed of conjunctions of literals of the forms $x=y\setminus z$, $x \neq y\setminus z$, and $z =\{x\}$, where $x,y,z$ stand for variables ranging over the von Neumann universe of sets, into unquantified Boolean formulae of a rather simple conjunctive normal form. The formulae in the target language involve variables ranging over a Boolean ring of sets, along with a difference operator and relators designating equality, non-disjointness and inclusion. Moreover, the result of each translation is a conjunction of literals of the forms $x=y\setminus z$, $x\neq y\setminus z$ and of implications whose antecedents are isolated literals and whose consequents are either inclusions (strict or non-strict) between variables, or equalities between variables. Besides reflecting a simple and natural semantics, which ensures satisfiability-preservation, the proposed translation has quadratic algorithmic time-complexity, and bridges two languages both of which are known to have an NP-complete satisfiability problem.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge