Controller Synthesis from Noisy-Input Noisy-Output Data

We consider the problem of synthesizing a dynamic output-feedback controller for a linear system, using solely input-output data corrupted by measurement noise. To handle input-output data, an auxiliary representation of the original system is introduced. By exploiting the structure of the auxiliary system, we design a controller that robustly stabilizes all possible systems consistent with data. Notably, we also provide a novel solution to extend the results to generic multi-input multi-output systems. The findings are illustrated by numerical examples.

Obstacle Avoidance via Hybrid Feedback

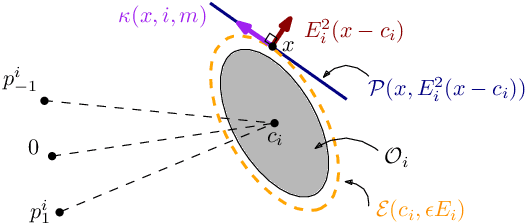
In this paper we present a hybrid feedback approach to solve the navigation problem of a point mass in the n-dimensional space containing an arbitrary number of ellipsoidal shape obstacles. The proposed hybrid control algorithm guarantees both global asymptotic stabilization to a reference and avoidance of the obstacles. The intuitive idea of the proposed hybrid feedback is to switch between two modes of control: stabilization and avoidance. The geometric construction of the flow and jump sets of the proposed hybrid controller, exploiting hysteresis regions, guarantees Zeno-free switching between the stabilization and the avoidance modes. Simulation results illustrate the performance of the proposed hybrid control approach for 2-dimensional and 3-dimensional scenarios.