Fast and Robust Structural Damage Analysis of Civil Infrastructure Using UAV Imagery

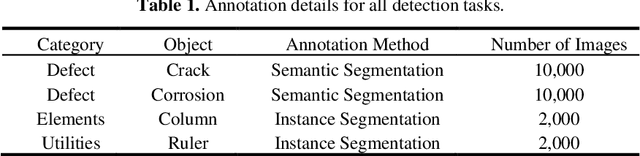
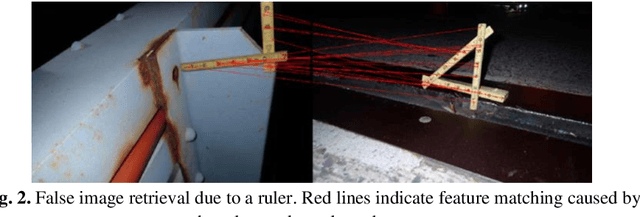
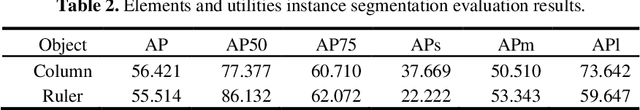
The usage of Unmanned Aerial Vehicles (UAVs) in the context of structural health inspection is recently gaining tremendous popularity. Camera mounted UAVs enable the fast acquisition of a large number of images often used for mapping, 3D model reconstruction, and as an assisting tool for inspectors. Due to the number of images captured during large scale UAV surveys, a manual image-based inspection analysis of entire assets cannot be efficiently performed by qualified engineers. Additionally, comparing defects to past inspections requires the retrieval of relevant images which is often impractical without extensive metadata or computer-vision-based algorithms. In this paper, we propose an end-to-end method for automated structural inspection damage analysis. Using automated object detection and segmentation we accurately localize defects, bridge utilities and elements. Next, given the high overlap in UAV imagery, points of interest are extracted, and defects are located and matched throughout the image database, considerably reducing data redundancy while maintaining a detailed record of the defects. Our technique not only enables fast and robust damage analysis of UAV imagery, as we show herein, but is also effective for analyzing manually acquired images.

Faithful Autoencoder Interpolation by Shaping the Latent Space

One of the fascinating properties of deep learning is the ability of the network to reveal the underlying factors characterizing elements in datasets of different types. Autoencoders represent an effective approach for computing these factors. Autoencoders have been studied in the context of their ability to interpolate between data points by decoding mixed latent vectors. However, this interpolation often incorporates disrupting artifacts or produces unrealistic images during reconstruction. We argue that these incongruities are due to the manifold structure of the latent space where interpolated latent vectors deviate from the data manifold. In this paper, we propose a regularization technique that shapes the latent space following the manifold assumption while enforcing the manifold to be smooth and convex. This regularization enables faithful interpolation between data points and can be used as a general regularization as well for avoiding overfitting and constraining the model complexity.