Recursive Learning of Asymptotic Variational Objectives

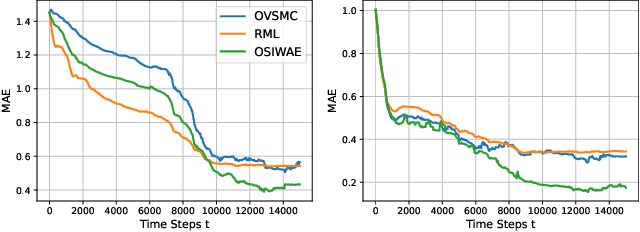
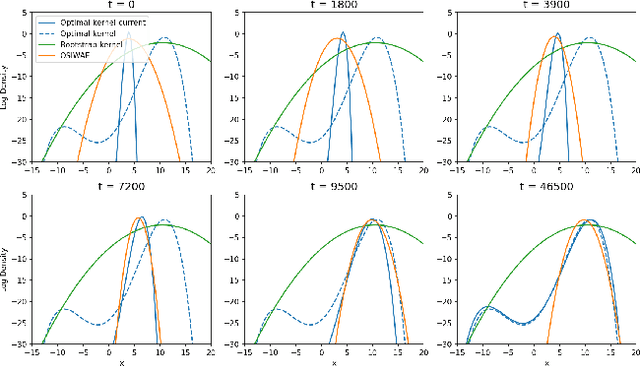
General state-space models (SSMs) are widely used in statistical machine learning and are among the most classical generative models for sequential time-series data. SSMs, comprising latent Markovian states, can be subjected to variational inference (VI), but standard VI methods like the importance-weighted autoencoder (IWAE) lack functionality for streaming data. To enable online VI in SSMs when the observations are received in real time, we propose maximising an IWAE-type variational lower bound on the asymptotic contrast function, rather than the standard IWAE ELBO, using stochastic approximation. Unlike the recursive maximum likelihood method, which directly maximises the asymptotic contrast, our approach, called online sequential IWAE (OSIWAE), allows for online learning of both model parameters and a Markovian recognition model for inferring latent states. By approximating filter state posteriors and their derivatives using sequential Monte Carlo (SMC) methods, we create a particle-based framework for online VI in SSMs. This approach is more theoretically well-founded than recently proposed online variational SMC methods. We provide rigorous theoretical results on the learning objective and a numerical study demonstrating the method's efficiency in learning model parameters and particle proposal kernels.

Online Variational Sequential Monte Carlo

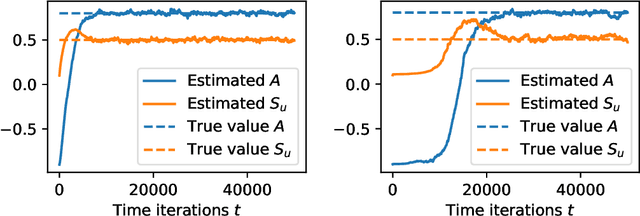
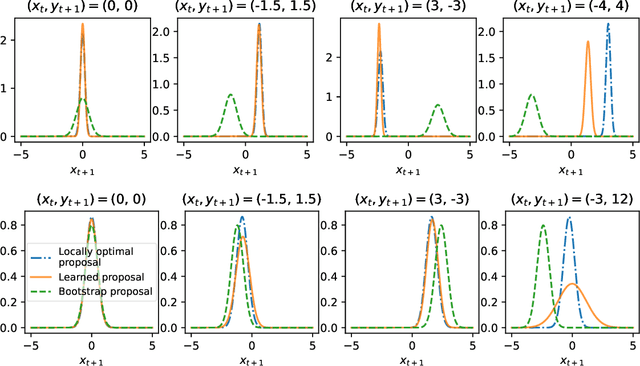
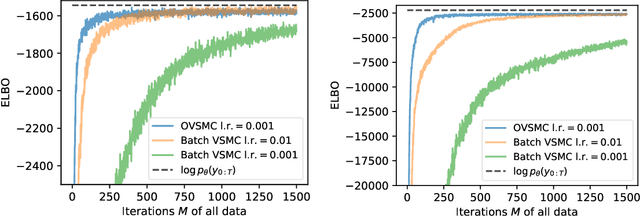
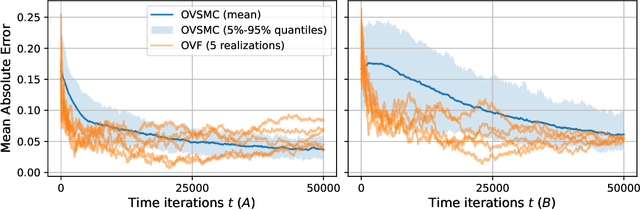
Being the most classical generative model for serial data, state-space models (SSM) are fundamental in AI and statistical machine learning. In SSM, any form of parameter learning or latent state inference typically involves the computation of complex latent-state posteriors. In this work, we build upon the variational sequential Monte Carlo (VSMC) method, which provides computationally efficient and accurate model parameter estimation and Bayesian latent-state inference by combining particle methods and variational inference. While standard VSMC operates in the offline mode, by re-processing repeatedly a given batch of data, we distribute the approximation of the gradient of the VSMC surrogate ELBO in time using stochastic approximation, allowing for online learning in the presence of streams of data. This results in an algorithm, online VSMC, that is capable of performing efficiently, entirely on-the-fly, both parameter estimation and particle proposal adaptation. In addition, we provide rigorous theoretical results describing the algorithm's convergence properties as the number of data tends to infinity as well as numerical illustrations of its excellent convergence properties and usefulness also in batch-processing settings.