Adam P. Generale
Conditional Variable Flow Matching: Transforming Conditional Densities with Amortized Conditional Optimal Transport
Nov 13, 2024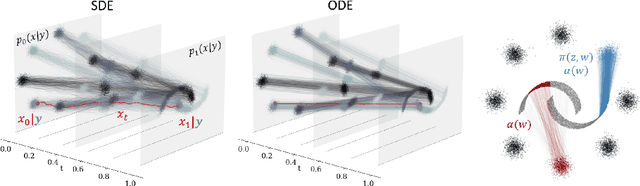
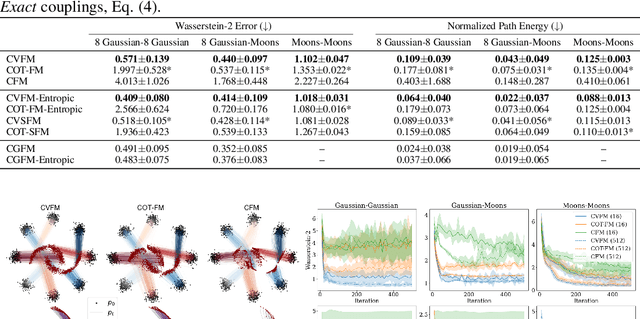
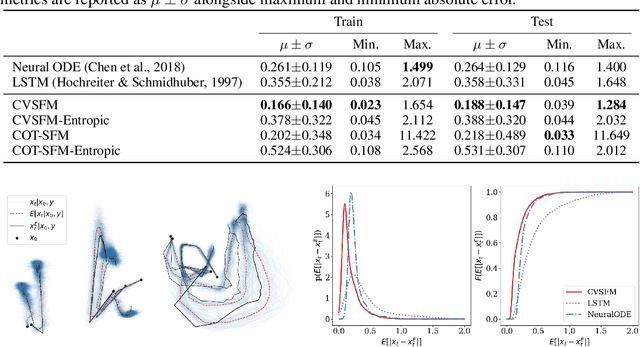
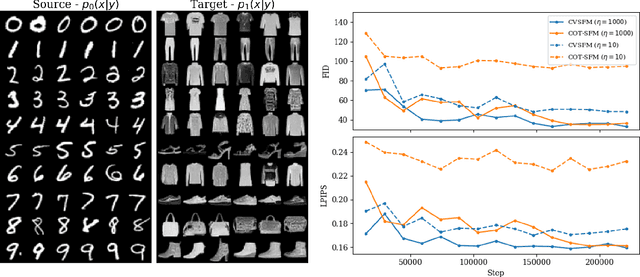
Abstract:Forecasting stochastic nonlinear dynamical systems under the influence of conditioning variables is a fundamental challenge repeatedly encountered across the biological and physical sciences. While flow-based models can impressively predict the temporal evolution of probability distributions representing possible outcomes of a specific process, existing frameworks cannot satisfactorily account for the impact of conditioning variables on these dynamics. Amongst several limitations, existing methods require training data with paired conditions and are developed for discrete conditioning variables. We propose Conditional Variable Flow Matching (CVFM), a framework for learning flows transforming conditional distributions with amortization across continuous conditioning variables - permitting predictions across the conditional density manifold. This is accomplished through several novel advances, in particular, simultaneous sample conditioned flows over the main and conditioning variables, alongside a conditional Wasserstein distance and kernel facilitating conditional optimal transport. Collectively, these advances allow for learning system dynamics provided measurement data whose states and conditioning variables are not in correspondence. We demonstrate CVFM on a suite of increasingly challenging problems, including discrete and continuous conditional mapping benchmarks, image-to-image domain transfer, and modeling the temporal evolution of materials internal structure during manufacturing processes. We observe that CVFM results in improved performance and convergence characteristics over alternative conditional variants.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge