Wasserstein total variation filtering
Paper and Code
Oct 23, 2019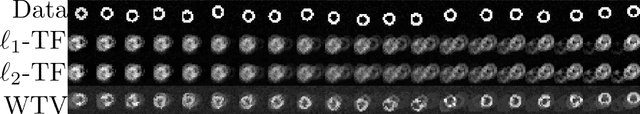

In this paper, we expand upon the theory of trend filtering by introducing the use of the Wasserstein metric as a means to control the amount of spatiotemporal variation in filtered time series data. While trend filtering utilizes regularization to produce signal estimates that are piecewise linear, in the case of $\ell_1$ regularization, or temporally smooth, in the case of $\ell_2$ regularization, it ignores the topology of the spatial distribution of signal. By incorporating the information about the underlying metric space of the pixel layout, the Wasserstein metric is an attractive choice as a regularizer to undercover spatiotemporal trends in time series data. We introduce a globally optimal algorithm for efficiently estimating the filtered signal under a Wasserstein finite differences operator. The efficacy of the proposed algorithm in preserving spatiotemporal trends in time series video is demonstrated in both simulated and fluorescent microscopy videos of the nematode caenorhabditis elegans and compared against standard trend filtering algorithms.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge