TriVoC: Efficient Voting-based Consensus Maximization for Robust Point Cloud Registration with Extreme Outlier Ratios
Paper and Code
Nov 01, 2021
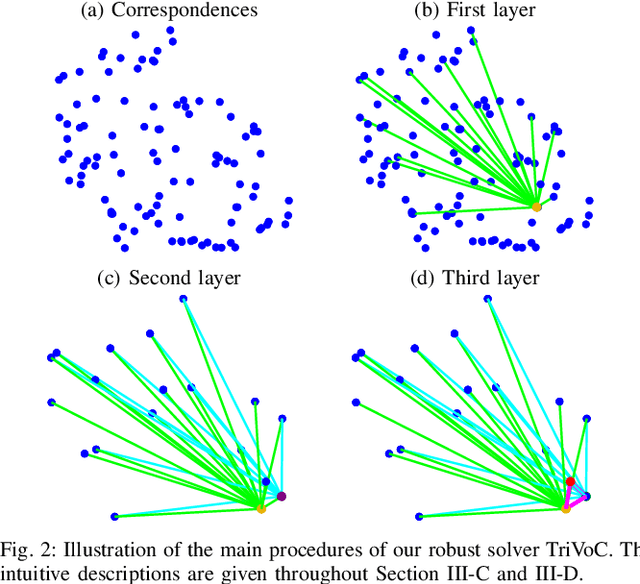
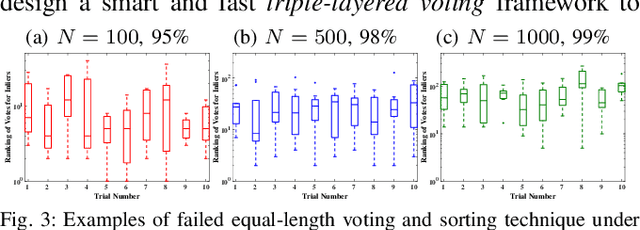
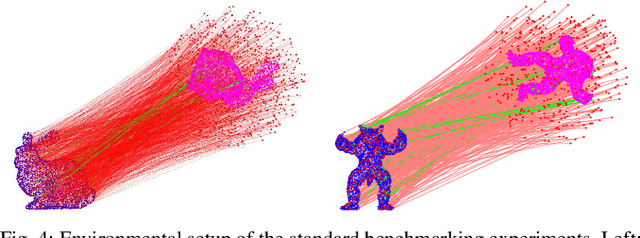
Correspondence-based point cloud registration is a cornerstone in robotics perception and computer vision, which seeks to estimate the best rigid transformation aligning two point clouds from the putative correspondences. However, due to the limited robustness of 3D keypoint matching approaches, outliers, probably in large numbers, are prone to exist among the correspondences, which makes robust registration methods imperative. Unfortunately, existing robust methods have their own limitations (e.g. high computational cost or limited robustness) when facing high or extreme outlier ratios, probably unsuitable for practical use. In this paper, we present a novel, fast, deterministic and guaranteed robust solver, named TriVoC (Triple-layered Voting with Consensus maximization), for the robust registration problem. We decompose the selecting of the minimal 3-point sets into 3 consecutive layers, and in each layer we design an efficient voting and correspondence sorting framework on the basis of the pairwise equal-length constraint. In this manner, the 3-point sets can be selected independently from the reduced correspondence sets according to the sorted sequence, which can significantly lower the computational cost and meanwhile provide a strong guarantee to achieve the largest consensus set (as the final inlier set) as long as a probabilistic termination condition is fulfilled. Varied experiments show that our solver TriVoC is robust against up to 99% outliers, highly accurate, time-efficient even with extreme outlier ratios, and also practical for real-world applications, showing performance superior to other state-of-the-art competitors.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge