Time-Varying Correlation Networks for Interpretable Change Point Detection
Paper and Code
Nov 08, 2022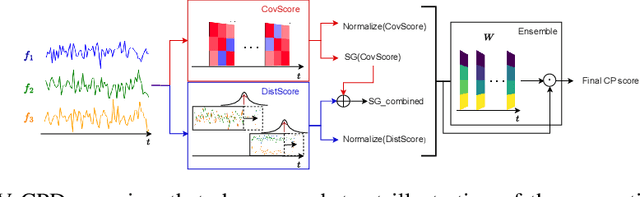
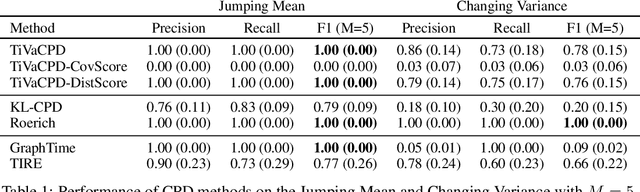
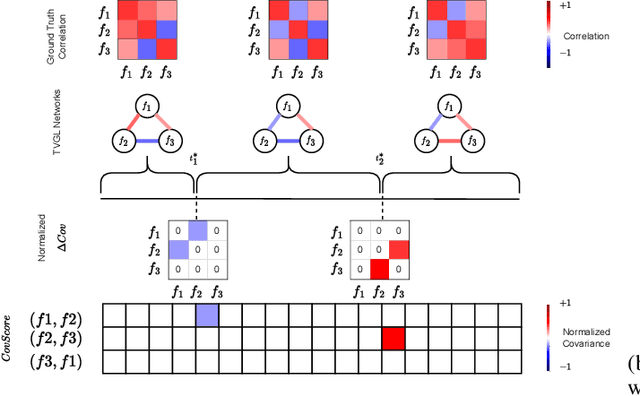
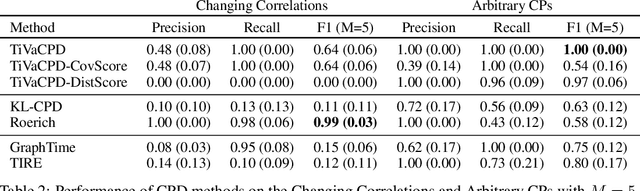
Change point detection (CPD) methods aim to detect abrupt changes in time-series data. Recent CPD methods have demonstrated their potential in identifying changes in underlying statistical distributions but often fail to capture complex changes in the correlation structure in time-series data. These methods also fail to generalize effectively, as even within the same time-series, different kinds of change points (CPs) may arise that are best characterized by different types of time-series perturbations. To address this issue, we propose TiVaCPD, a CPD methodology that uses a time-varying graphical lasso based method to identify changes in correlation patterns between features over time, and combines that with an aggregate Kernel Maximum Mean Discrepancy (MMD) test to identify subtle changes in the underlying statistical distributions of dynamically established time windows. We evaluate the performance of TiVaCPD in identifying and characterizing various types of CPs in time-series and show that our method outperforms current state-of-the-art CPD methods for all categories of CPs.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge