The Gaussian Transform
Paper and Code
Jun 21, 2020
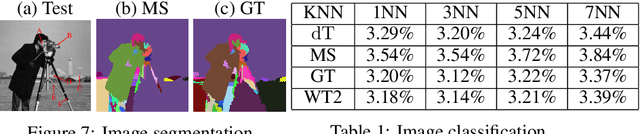
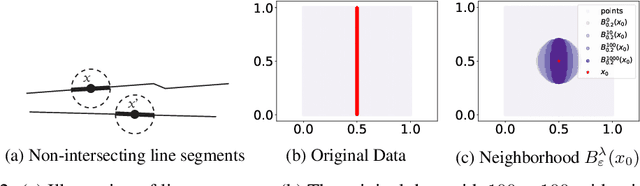
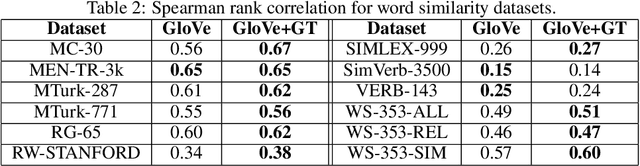
We introduce the Gaussian transform (GT), an optimal transport inspired iterative method for denoising and enhancing latent structures in datasets. Under the hood, GT generates a new distance function (GT distance) on a given dataset by computing the $\ell^2$-Wasserstein distance between certain Gaussian density estimates obtained by localizing the dataset to individual points. Our contribution is twofold: (1) theoretically, we establish firstly that GT is stable under perturbations and secondly that in the continuous case, each point possesses an asymptotically ellipsoidal neighborhood with respect to the GT distance; (2) computationally, we accelerate GT both by identifying a strategy for reducing the number of matrix square root computations inherent to the $\ell^2$-Wasserstein distance between Gaussian measures, and by avoiding redundant computations of GT distances between points via enhanced neighborhood mechanisms. We also observe that GT is both a generalization and a strengthening of the mean shift (MS) method, and it is also a computationally efficient specialization of the recently proposed Wasserstein Transform (WT) method. We perform extensive experimentation comparing their performance in different scenarios.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge