Testing to distinguish measures on metric spaces
Paper and Code
Feb 04, 2018
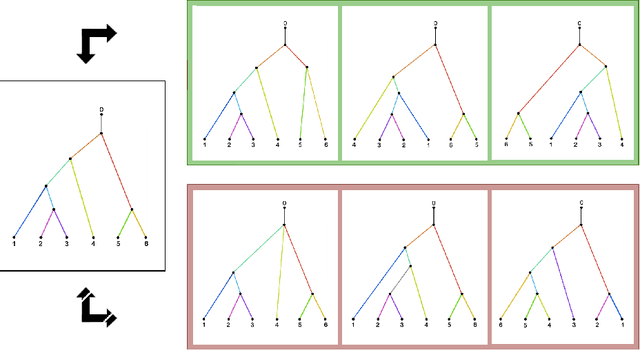
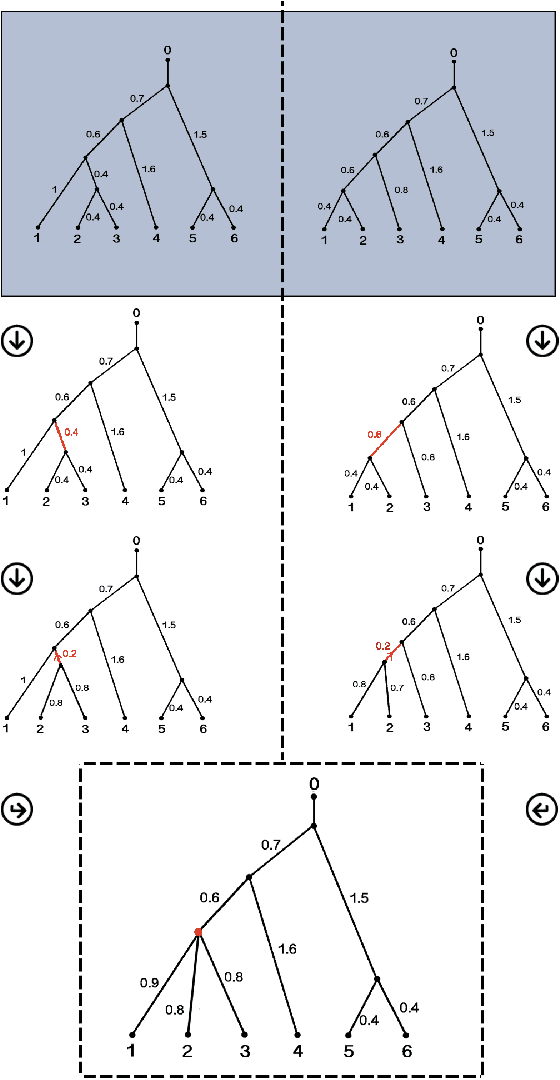
We study the problem of distinguishing between two distributions on a metric space; i.e., given metric measure spaces $({\mathbb X}, d, \mu_1)$ and $({\mathbb X}, d, \mu_2)$, we are interested in the problem of determining from finite data whether or not $\mu_1$ is $\mu_2$. The key is to use pairwise distances between observations and, employing a reconstruction theorem of Gromov, we can perform such a test using a two sample Kolmogorov--Smirnov test. A real analysis using phylogenetic trees and flu data is presented.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge