SC-MAD: Mixtures of Higher-order Networks for Data Augmentation
Paper and Code
Sep 14, 2023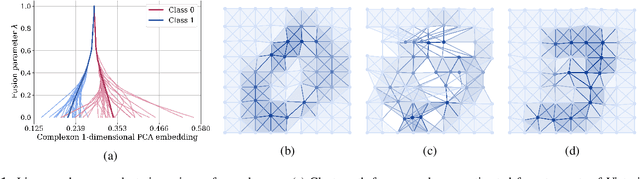
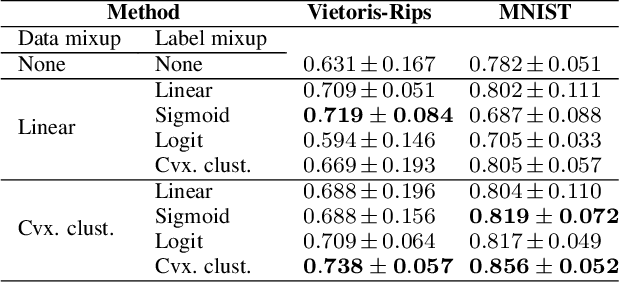
The myriad complex systems with multiway interactions motivate the extension of graph-based pairwise connections to higher-order relations. In particular, the simplicial complex has inspired generalizations of graph neural networks (GNNs) to simplicial complex-based models. Learning on such systems requires large amounts of data, which can be expensive or impossible to obtain. We propose data augmentation of simplicial complexes through both linear and nonlinear mixup mechanisms that return mixtures of existing labeled samples. In addition to traditional pairwise mixup, we present a convex clustering mixup approach for a data-driven relationship among several simplicial complexes. We theoretically demonstrate that the resultant synthetic simplicial complexes interpolate among existing data with respect to homomorphism densities. Our method is demonstrated on both synthetic and real-world datasets for simplicial complex classification.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge