Restricted Boltzmann Stochastic Block Model: A Generative Model for Networks with Attributes

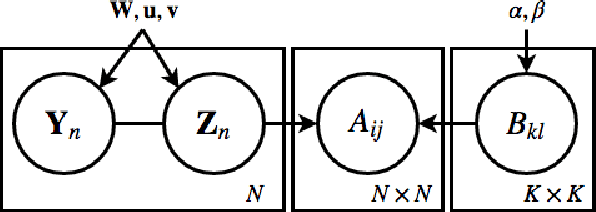
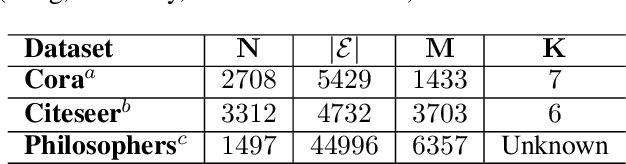
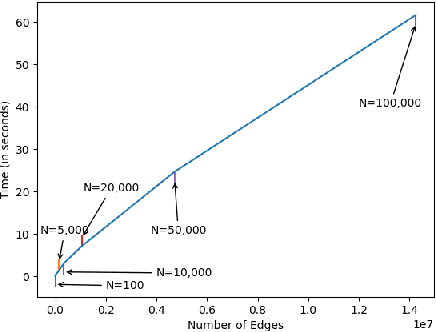
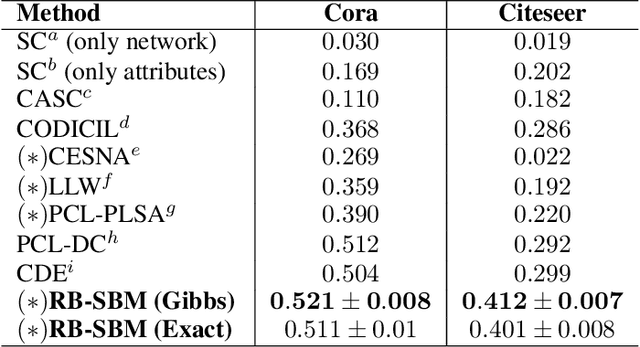
In most practical contexts network indexed data consists not only of a description about the presence/absence of links, but also attributes and information about the nodes and/or links. Building on success of Stochastic Block Models (SBM) we propose a simple yet powerful generalization of SBM for networks with node attributes. In a standard SBM the rows of latent community membership matrix are sampled from a multinomial. In RB-SBM, our proposed model, these rows are sampled from a Restricted Boltzmann Machine (RBM) that models a joint distribution over observed attributes and latent community membership. This model has the advantage of being simple while combining connectivity and attribute information, and it has very few tuning parameters. Furthermore, we show that inference can be done efficiently in linear time and it can be naturally extended to accommodate, for instance, overlapping communities. We demonstrate the performance of our model on multiple synthetic and real world networks with node attributes where we obtain state-of-the-art results on the task of community detection.