Relaxing the Feature Covariance Assumption: Time-Variant Bounds for Benign Overfitting in Linear Regression
Paper and Code
Feb 12, 2022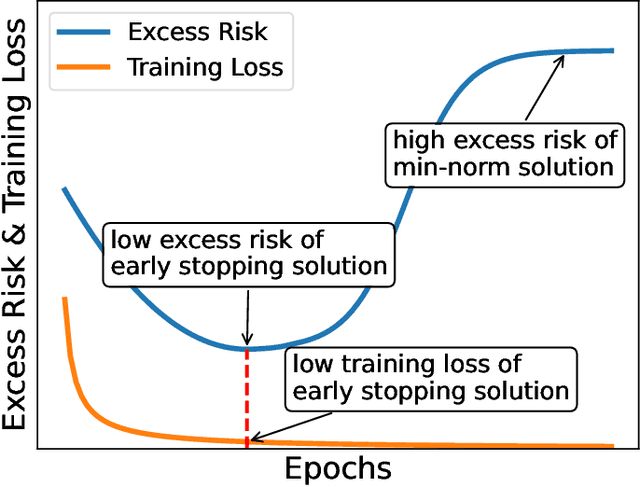
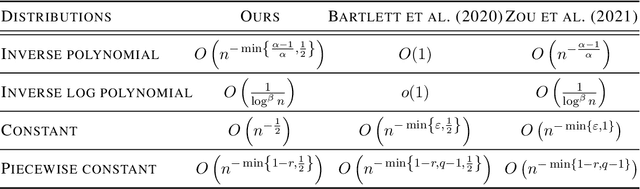
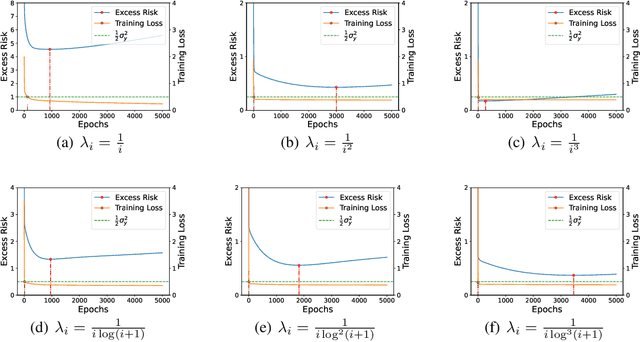
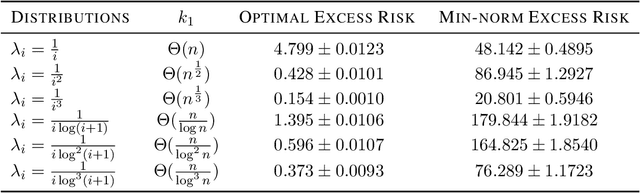
Benign overfitting demonstrates that overparameterized models can perform well on test data while fitting noisy training data. However, it only considers the final min-norm solution in linear regression, which ignores the algorithm information and the corresponding training procedure. In this paper, we generalize the idea of benign overfitting to the whole training trajectory instead of the min-norm solution and derive a time-variant bound based on the trajectory analysis. Starting from the time-variant bound, we further derive a time interval that suffices to guarantee a consistent generalization error for a given feature covariance. Unlike existing approaches, the newly proposed generalization bound is characterized by a time-variant effective dimension of feature covariance. By introducing the time factor, we relax the strict assumption on the feature covariance matrix required in previous benign overfitting under the regimes of overparameterized linear regression with gradient descent. This paper extends the scope of benign overfitting, and experiment results indicate that the proposed bound accords better with empirical evidence.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge