Reflected Schrödinger Bridge: Density Control with Path Constraints

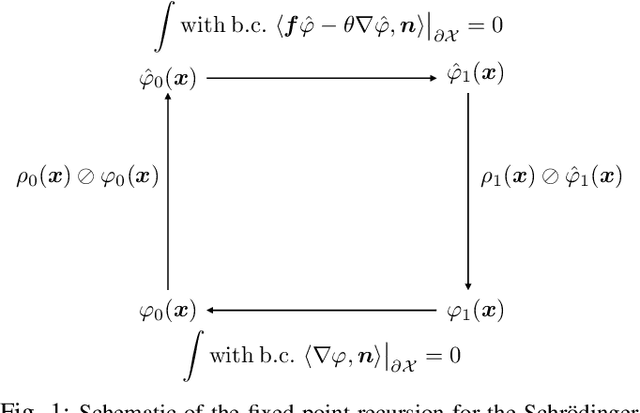
How to steer a given joint state probability density function to another over finite horizon subject to a controlled stochastic dynamics with hard state (sample path) constraints? In applications, state constraints may encode safety requirements such as obstacle avoidance. In this paper, we perform the feedback synthesis for minimum control effort density steering (a.k.a. Schr\"{o}dinger bridge) problem subject to state constraints. We extend the theory of Schr\"{o}dinger bridges to account the reflecting boundary conditions for the sample paths, and provide a computational framework building on our previous work on proximal recursions, to solve the same.