Reducing the Control Overhead of Intelligent Reconfigurable Surfaces Via a Tensor-Based Low-Rank Factorization Approach
Paper and Code
Jun 10, 2022
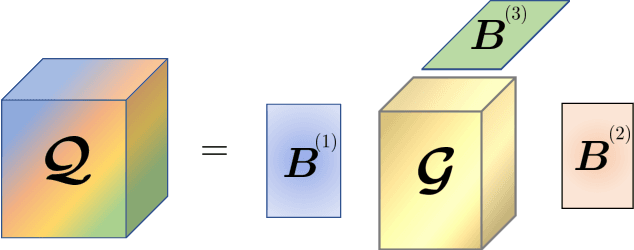

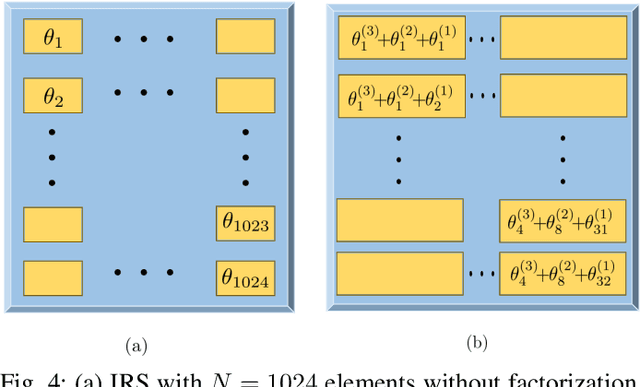
Passive intelligent reconfigurable surfaces (IRS) are becoming an attractive component of cellular networks due to their ability of shaping the propagation environment and thereby improving the coverage. While passive IRS nodes incorporate a great number of phase-shifting elements and a controller entity, the phase-shifts are typically determined by the cellular base station (BS) due to its computational capability. Since the fine granularity control of the large number of phase-shifters may become prohibitive in practice, it is important to reduce the control overhead between the BS and the IRS controller. To this end, in this paper we propose a low-rank approximation of the near-optimal phase-shifts, which would incur prohibitively high communication overhead on the BS-IRS controller links. The key idea is to represent the potentially large IRS phase-shift vector using a low-rank tensor model. This is achieved by factorizing a tensorized version of the IRS phase-shift vector, where each component is modeled as the Kronecker product of a predefined number of factors of smaller sizes, which can be obtained via tensor decomposition algorithms. We show that the proposed low-rank models drastically reduce the required feedback requirements associated with the BS-IRS control links. Our simulation results indicate that the proposed method is especially attractive in scenarios with a strong line of sight component, in which case nearly the same spectral efficiency is reached as in the cases with near-optimal phase-shifts, but with a drastically reduced communication overhead.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge