Recycling Computed Answers in Rewrite Systems for Abduction

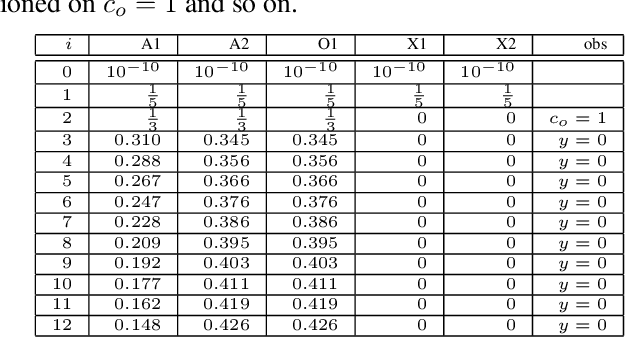
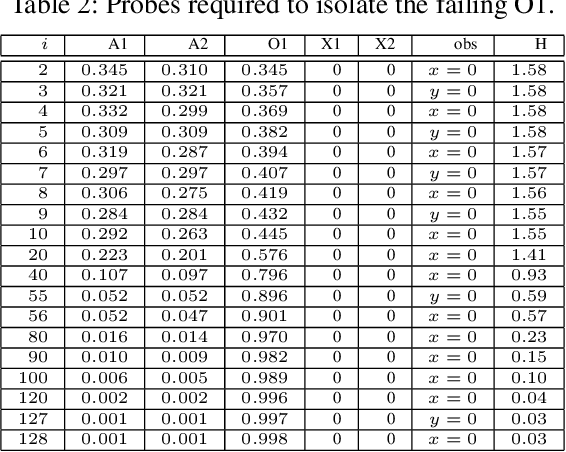
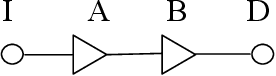
In rule-based systems, goal-oriented computations correspond naturally to the possible ways that an observation may be explained. In some applications, we need to compute explanations for a series of observations with the same domain. The question whether previously computed answers can be recycled arises. A yes answer could result in substantial savings of repeated computations. For systems based on classic logic, the answer is YES. For nonmonotonic systems however, one tends to believe that the answer should be NO, since recycling is a form of adding information. In this paper, we show that computed answers can always be recycled, in a nontrivial way, for the class of rewrite procedures that we proposed earlier for logic programs with negation. We present some experimental results on an encoding of the logistics domain.