Recursive Two-Step Lookahead Expected Payoff for Time-Dependent Bayesian Optimization
Paper and Code
Jun 14, 2020
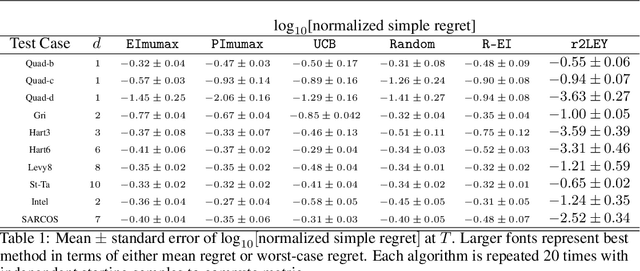


We propose a novel Bayesian method to solve the maximization of a time-dependent expensive-to-evaluate oracle. We are interested in the decision that maximizes the oracle at a finite time horizon, when relatively few noisy evaluations can be performed before the horizon. Our recursive, two-step lookahead expected payoff ($\texttt{r2LEY}$) acquisition function makes nonmyopic decisions at every stage by maximizing the estimated expected value of the oracle at the horizon. $\texttt{r2LEY}$ circumvents the evaluation of the expensive multistep (more than two steps) lookahead acquisition function by recursively optimizing a two-step lookahead acquisition function at every stage; unbiased estimators of this latter function and its gradient are utilized for efficient optimization. $\texttt{r2LEY}$ is shown to exhibit natural exploration properties far from the time horizon, enabling accurate emulation of the oracle, which is exploited in the final decision made at the horizon. To demonstrate the utility of $\texttt{r2LEY}$, we compare it with time-dependent extensions of popular myopic acquisition functions via both synthetic and real-world datasets.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge