Quasi-Newton's method in the class gradient defined high-curvature subspace
Paper and Code
Nov 28, 2020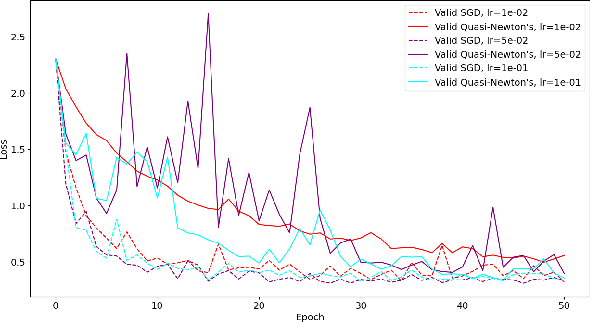
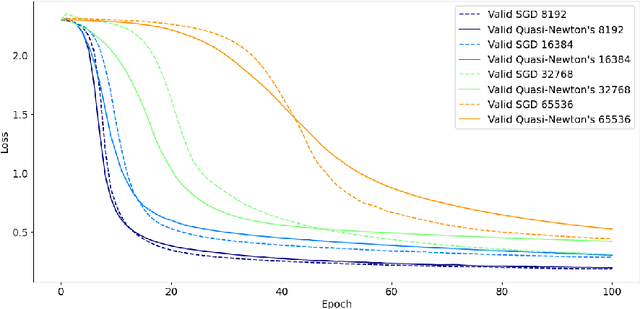
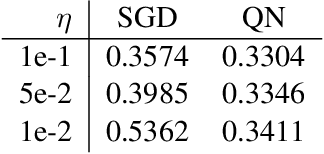
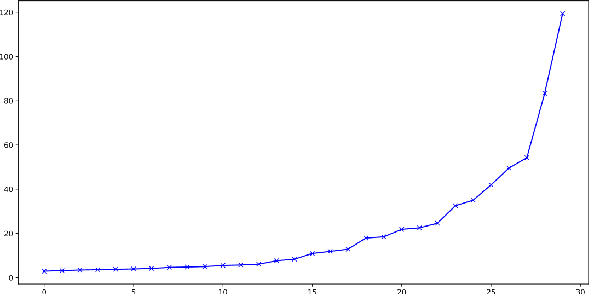
Classification problems using deep learning have been shown to have a high-curvature subspace in the loss landscape equal in dimension to the number of classes. Moreover, this subspace corresponds to the subspace spanned by the logit gradients for each class. An obvious strategy to speed up optimisation would be to use Newton's method in the high-curvature subspace and stochastic gradient descent in the co-space. We show that a naive implementation actually slows down convergence and we speculate why this might be.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge