Quantum Geometric Machine Learning for Quantum Circuits and Control
Paper and Code
Jul 07, 2020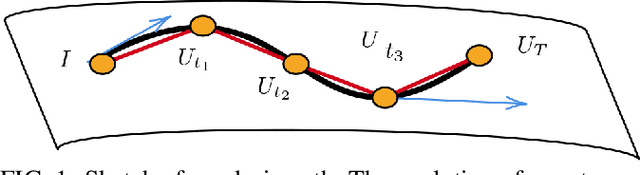
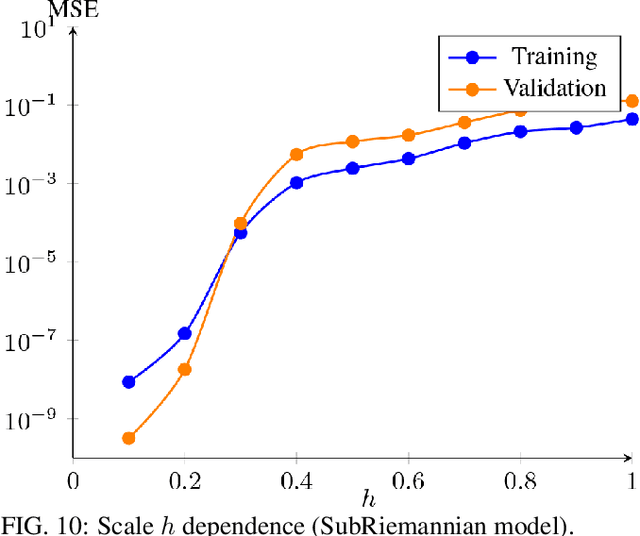
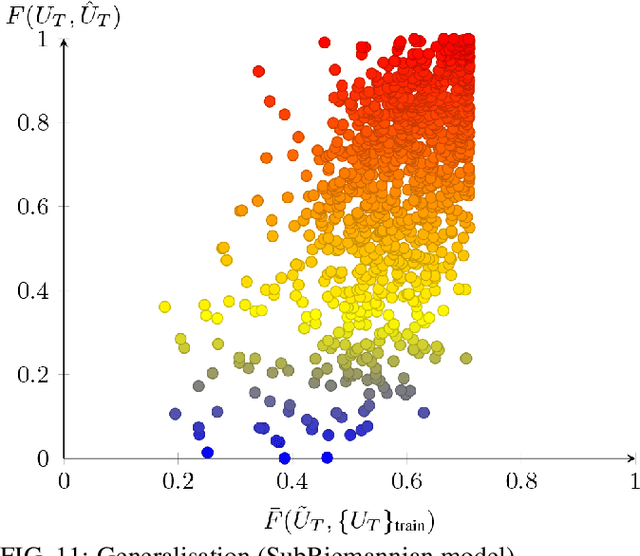
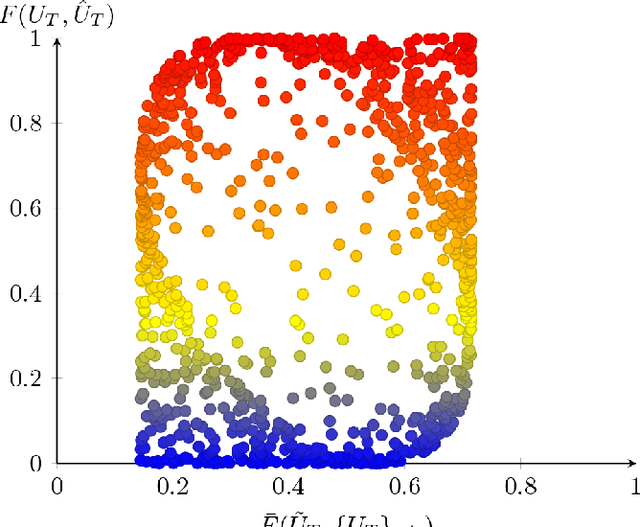
The application of machine learning techniques to solve problems in quantum control together with established geometric methods for solving optimisation problems leads naturally to an exploration of how machine learning approaches can be used to enhance geometric approaches to solving problems in quantum information processing. In this work, we review and extend the application of deep learning to quantum geometric control problems. Specifically, we demonstrate enhancements in time-optimal control in the context of quantum circuit synthesis problems by applying novel deep learning algorithms in order to approximate geodesics (and thus minimal circuits) along Lie group manifolds relevant to low-dimensional multi-qubit systems, such as SU(2), SU(4) and SU(8). We demonstrate the superior performance of greybox models, which combine traditional blackbox algorithms with prior domain knowledge of quantum mechanics, as means of learning underlying quantum circuit distributions of interest. Our results demonstrate how geometric control techniques can be used to both (a) verify the extent to which geometrically synthesised quantum circuits lie along geodesic, and thus time-optimal, routes and (b) synthesise those circuits. Our results are of interest to researchers in quantum control and quantum information theory seeking to combine machine learning and geometric techniques for time-optimal control problems.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge