Quantum Boosting using Domain-Partitioning Hypotheses

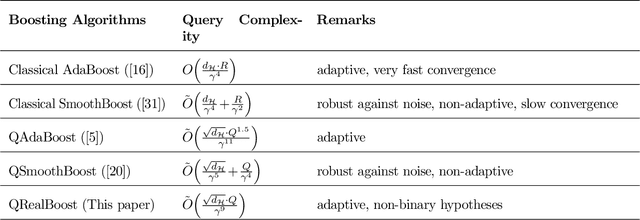
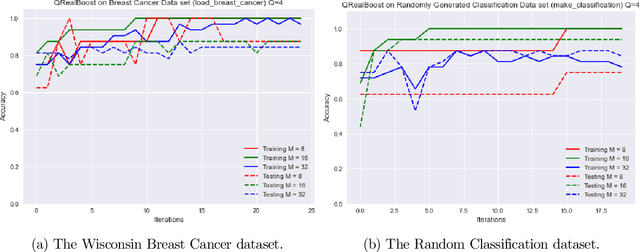
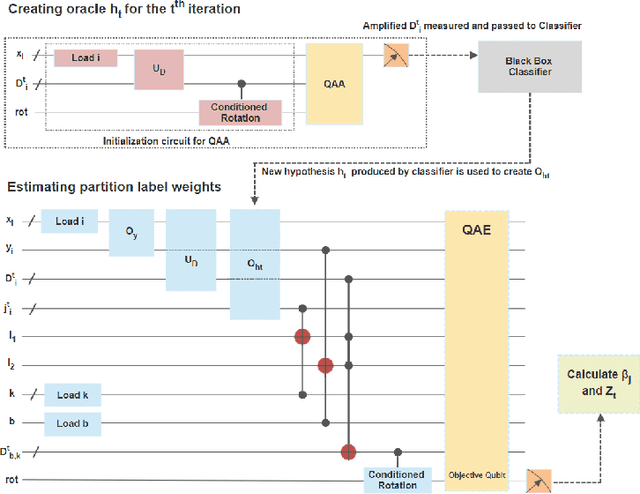
Boosting is an ensemble learning method that converts a weak learner into a strong learner in the PAC learning framework. Freund and Schapire gave the first classical boosting algorithm for binary hypothesis known as AdaBoost, and this was recently adapted into a quantum boosting algorithm by Arunachalam et al. Their quantum boosting algorithm (which we refer to as Q-AdaBoost) is quadratically faster than the classical version in terms of the VC-dimension of the hypothesis class of the weak learner but polynomially worse in the bias of the weak learner. In this work we design a different quantum boosting algorithm that uses domain partitioning hypotheses that are significantly more flexible than those used in prior quantum boosting algorithms in terms of margin calculations. Our algorithm Q-RealBoost is inspired by the "Real AdaBoost" (aka. RealBoost) extension to the original AdaBoost algorithm. Further, we show that Q-RealBoost provides a polynomial speedup over Q-AdaBoost in terms of both the bias of the weak learner and the time taken by the weak learner to learn the target concept class.