Posterior Sampling for Image Restoration using Explicit Patch Priors
Paper and Code
Apr 20, 2021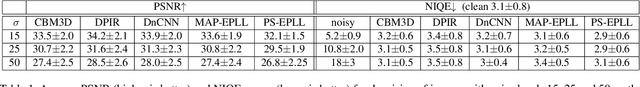
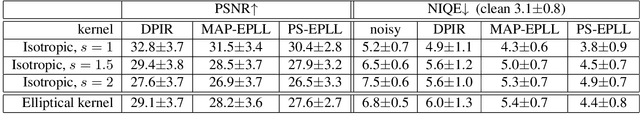

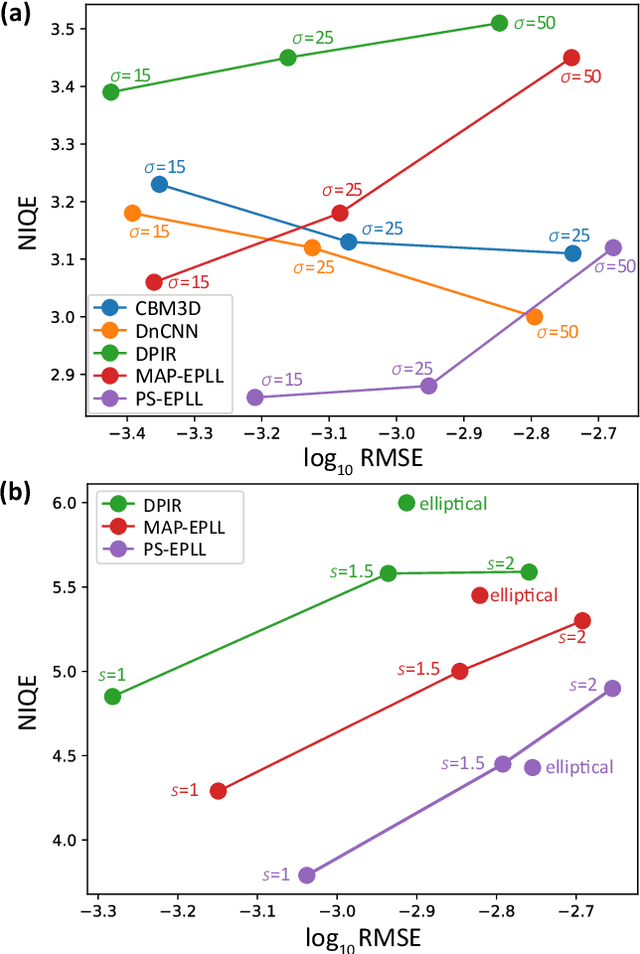
Almost all existing methods for image restoration are based on optimizing the mean squared error (MSE), even though it is known that the best estimate in terms of MSE may yield a highly atypical image due to the fact that there are many plausible restorations for a given noisy image. In this paper, we show how to combine explicit priors on patches of natural images in order to sample from the posterior probability of a full image given a degraded image. We prove that our algorithm generates correct samples from the distribution $p(x|y) \propto \exp(-E(x|y))$ where $E(x|y)$ is the cost function minimized in previous patch-based approaches that compute a single restoration. Unlike previous approaches that computed a single restoration using MAP or MMSE, our method makes explicit the uncertainty in the restored images and guarantees that all patches in the restored images will be typical given the patch prior. Unlike previous approaches that used implicit priors on fixed-size images, our approach can be used with images of any size. Our experimental results show that posterior sampling using patch priors yields images of high perceptual quality and high PSNR on a range of challenging image restoration problems.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge