Participatory Budgeting with Project Groups
Paper and Code
Dec 09, 2020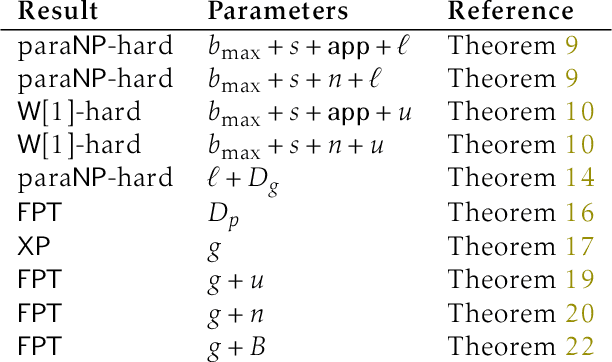

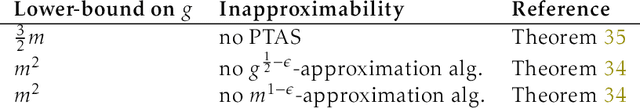
We study a generalization of the standard approval-based model of participatory budgeting (PB), in which voters are providing approval ballots over a set of predefined projects and -- in addition to a global budget limit, there are several groupings of the projects, each group with its own budget limit. We study the computational complexity of identifying project bundles that maximize voter satisfaction while respecting all budget limits. We show that the problem is generally intractable and describe efficient exact algorithms for several special cases, including instances with only few groups and instances where the group structure is close to be hierarchical, as well as efficient approximation algorithms. Our results could allow, e.g., municipalities to hold richer PB processes that are thematically and geographically inclusive.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge