Orthogonal symmetric non-negative matrix factorization under the stochastic block model
Paper and Code
May 17, 2016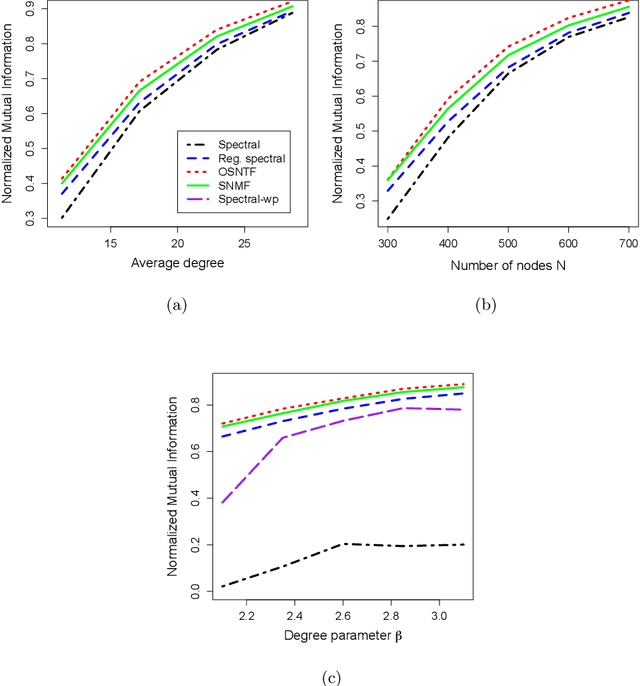
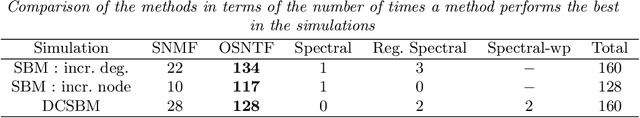

We present a method based on the orthogonal symmetric non-negative matrix tri-factorization of the normalized Laplacian matrix for community detection in complex networks. While the exact factorization of a given order may not exist and is NP hard to compute, we obtain an approximate factorization by solving an optimization problem. We establish the connection of the factors obtained through the factorization to a non-negative basis of an invariant subspace of the estimated matrix, drawing parallel with the spectral clustering. Using such factorization for clustering in networks is motivated by analyzing a block-diagonal Laplacian matrix with the blocks representing the connected components of a graph. The method is shown to be consistent for community detection in graphs generated from the stochastic block model and the degree corrected stochastic block model. Simulation results and real data analysis show the effectiveness of these methods under a wide variety of situations, including sparse and highly heterogeneous graphs where the usual spectral clustering is known to fail. Our method also performs better than the state of the art in popular benchmark network datasets, e.g., the political web blogs and the karate club data.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge