Optimal sequential decision making with probabilistic digital twins
Paper and Code
Mar 12, 2021

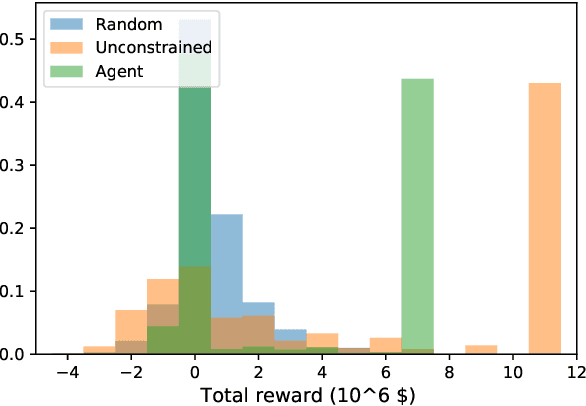
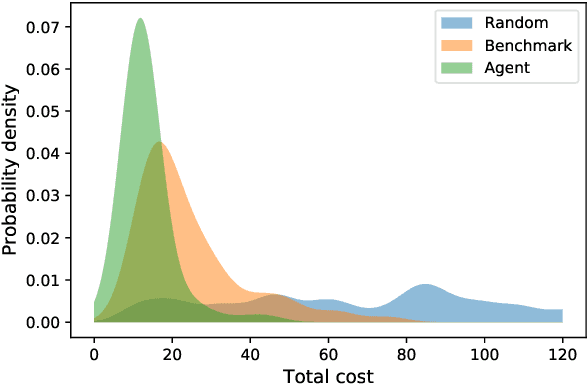
Digital twins are emerging in many industries, typically consisting of simulation models and data associated with a specific physical system. One of the main reasons for developing a digital twin, is to enable the simulation of possible consequences of a given action, without the need to interfere with the physical system itself. Physical systems of interest, and the environments they operate in, do not always behave deterministically. Moreover, information about the system and its environment is typically incomplete or imperfect. Probabilistic representations of systems and environments may therefore be called for, especially to support decisions in application areas where actions may have severe consequences. In this paper we introduce the probabilistic digital twin (PDT). We will start by discussing how epistemic uncertainty can be treated using measure theory, by modelling epistemic information via $\sigma$-algebras. Based on this, we give a formal definition of how epistemic uncertainty can be updated in a PDT. We then study the problem of optimal sequential decision making. That is, we consider the case where the outcome of each decision may inform the next. Within the PDT framework, we formulate this optimization problem. We discuss how this problem may be solved (at least in theory) via the maximum principle method or the dynamic programming principle. However, due to the curse of dimensionality, these methods are often not tractable in practice. To mend this, we propose a generic approximate solution using deep reinforcement learning together with neural networks defined on sets. We illustrate the method on a practical problem, considering optimal information gathering for the estimation of a failure probability.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge