Minimizing AoI in Resource-Constrained Multi-Source Relaying Systems with Stochastic Arrivals
Paper and Code
Sep 10, 2021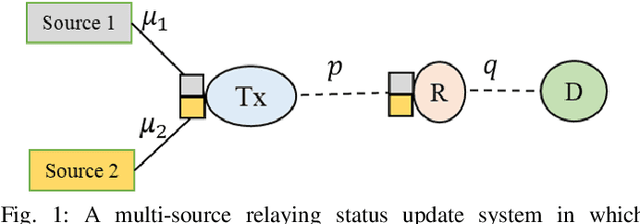
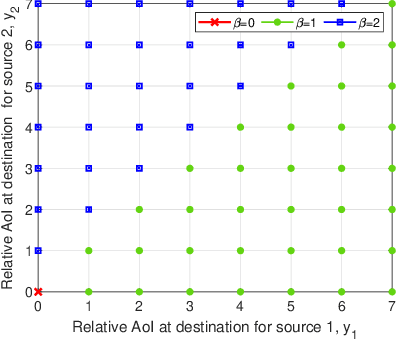
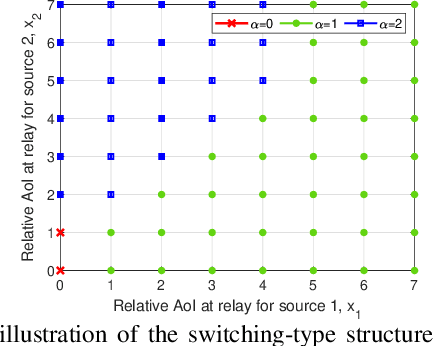

We consider a multi-source relaying system where the sources independently and randomly generate status update packets which are sent to the destination with the aid of a bufferaided relay through unreliable links. We formulate a stochastic optimization problem aiming to minimize the sum average age of information (AAoI) of sources under per-slot transmission capacity constraints and a long-run average resource constraint. To solve the problem, we recast it as a constrained Markov decision process (CMDP) problem and adopt the Lagrangian method. We analyze the structure of an optimal policy for the resulting MDP problem that possesses a switching-type structure. We propose an algorithm that obtains a stationary deterministic near-optimal policy, establishing a benchmark for the system. Simulation results show the effectiveness of our algorithm compared to benchmark algorithms.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge