Joint Learning of Linear Time-Invariant Dynamical Systems

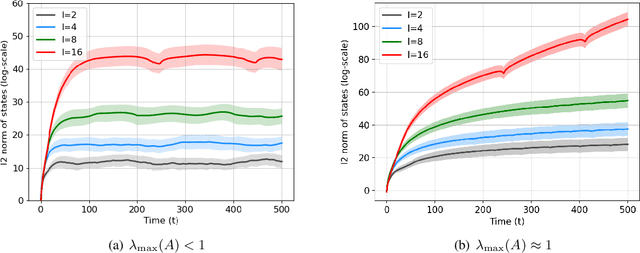
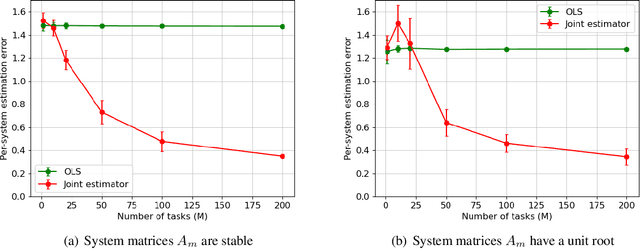
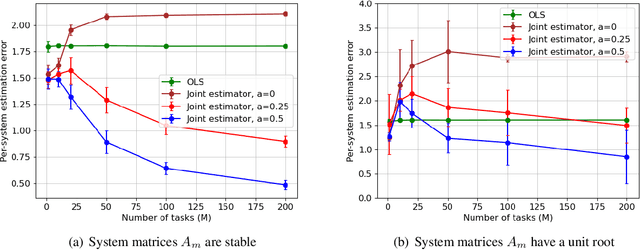
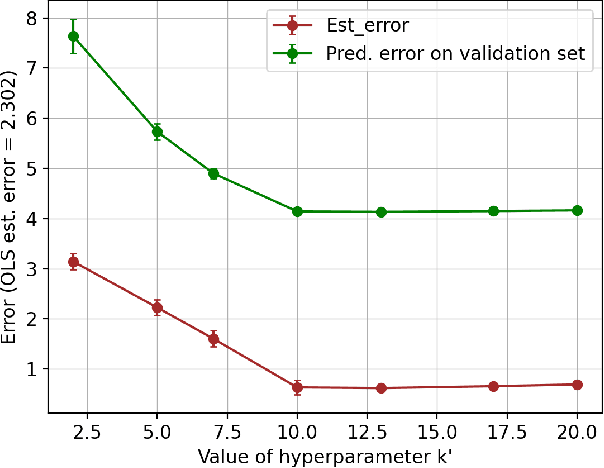
Learning the parameters of a linear time-invariant dynamical system (LTIDS) is a problem of current interest. In many applications, one is interested in jointly learning the parameters of multiple related LTIDS, which remains unexplored to date. To that end, we develop a joint estimator for learning the transition matrices of LTIDS that share common basis matrices. Further, we establish finite-time error bounds that depend on the underlying sample size, dimension, number of tasks, and spectral properties of the transition matrices. The results are obtained under mild regularity assumptions and showcase the gains from pooling information across LTIDS, in comparison to learning each system separately. We also study the impact of misspecifying the joint structure of the transition matrices and show that the established results are robust in the presence of moderate misspecifications.