Improved Bandits in Many-to-one Matching Markets with Incentive Compatibility

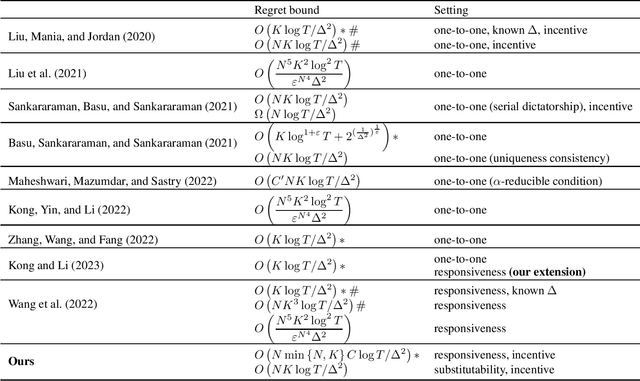
Two-sided matching markets have been widely studied in the literature due to their rich applications. Since participants are usually uncertain about their preferences, online algorithms have recently been adopted to learn them through iterative interactions. \citet{wang2022bandit} initiate the study of this problem in a many-to-one setting with \textit{responsiveness}. However, their results are far from optimal and lack guarantees of incentive compatibility. An extension of \citet{kong2023player} to this more general setting achieves a near-optimal bound for player-optimal regret. Nevertheless, due to the substantial requirement for collaboration, a single player's deviation could lead to a huge increase in its own cumulative rewards and an $O(T)$ regret for others. In this paper, we aim to enhance the regret bound in many-to-one markets while ensuring incentive compatibility. We first propose the adaptively explore-then-deferred-acceptance (AETDA) algorithm for responsiveness setting and derive an $O(N\min\left\{N,K\right\}C\log T/\Delta^2)$ upper bound for player-optimal stable regret while demonstrating its guarantee of incentive compatibility, where $N$ represents the number of players, $K$ is the number of arms, $T$ denotes the time horizon, $C$ is arms' total capacities and $\Delta$ signifies the minimum preference gap among players. This result is a significant improvement over \citet{wang2022bandit}. And to the best of our knowledge, it constitutes the first player-optimal guarantee in matching markets that offers such robust assurances. We also consider broader \textit{substitutable} preferences, one of the most general conditions to ensure the existence of a stable matching and cover responsiveness. We devise an online DA (ODA) algorithm and establish an $O(NK\log T/\Delta^2)$ player-pessimal stable regret bound for this setting.