Identification and Estimation of Hierarchical Latent Attribute Models

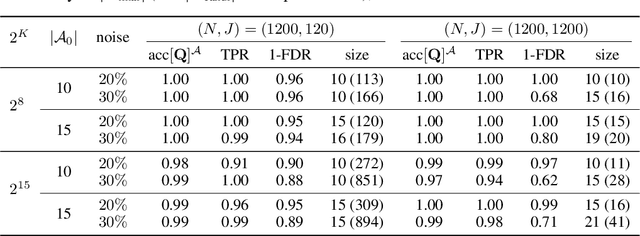
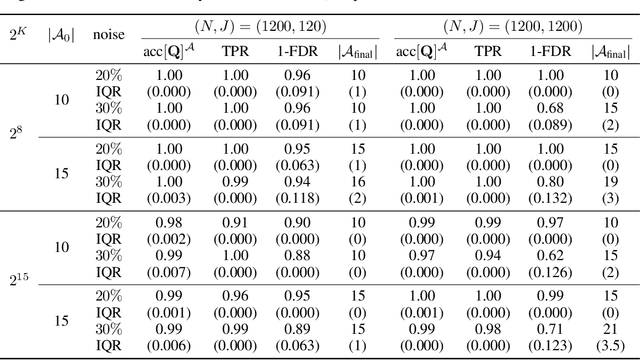
Hierarchical Latent Attribute Models (HLAMs) are a popular family of discrete latent variable models widely used in social and biological sciences. The key ingredients of an HLAM include a binary structural matrix specifying how the observed variables depend on the latent attributes, and also certain hierarchical constraints on allowable configurations of the latent attributes. This paper studies the theoretical identifiability issue and the practical estimation problem of HLAMs. For identification, the challenging problem of identifiability under a complex hierarchy is addressed and sufficient and almost necessary identification conditions are proposed. For estimation, a scalable algorithm for estimating both the structural matrix and the attribute hierarchy is developed. The superior performance of the proposed algorithm is demonstrated in various experimental settings, including both synthetic data and a real dataset from an international educational assessment.